(Solved): A notch is cut in a cylindrical vertical tree trunk. The notch penetrates to the axis o ...

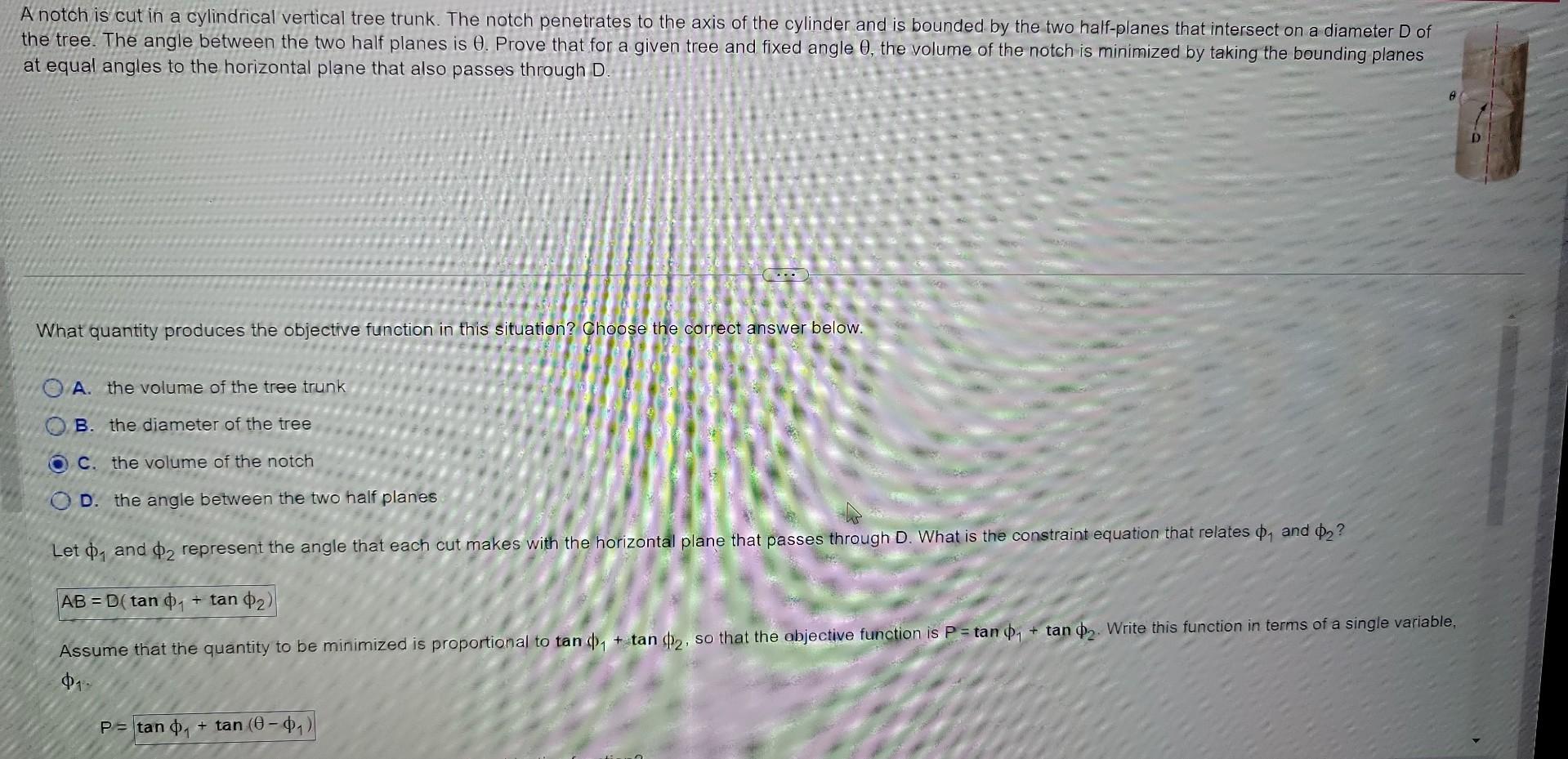
A notch is cut in a cylindrical vertical tree trunk. The notch penetrates to the axis of the cylinder and is bounded by the two half-planes that intersect on a diameter D of the tree. The angle between the two half planes is $?$. Prove that for a given tree and fixed angle $?$, the volume of the notch is minimized by taking the bounding planes at equal angles to the horizontal plane that also passes through $D$. What quantity produces the objective function in this situation? Choose the correct answer below. A. the volume of the tree trunk B. the diameter of the tree C. the volume of the notch D. the angle between the two half planes Let ${?}_1$ and ${?}_2$ represent the angle that each cut makes with the horizontal plane that passes through $\mathrm{D}$. What is the constraint equation that relates ${?}_1$ and ${?}_2$ ? $AB=D\left(\tan {?}_1+\tan {?}_2\right)$ Assume that the quantity to be minimized is proportional to $\tan {?}_1+\tan {?}_2$, so that the abjective function is $P=\tan {?}_1+\tan {?}_2$. 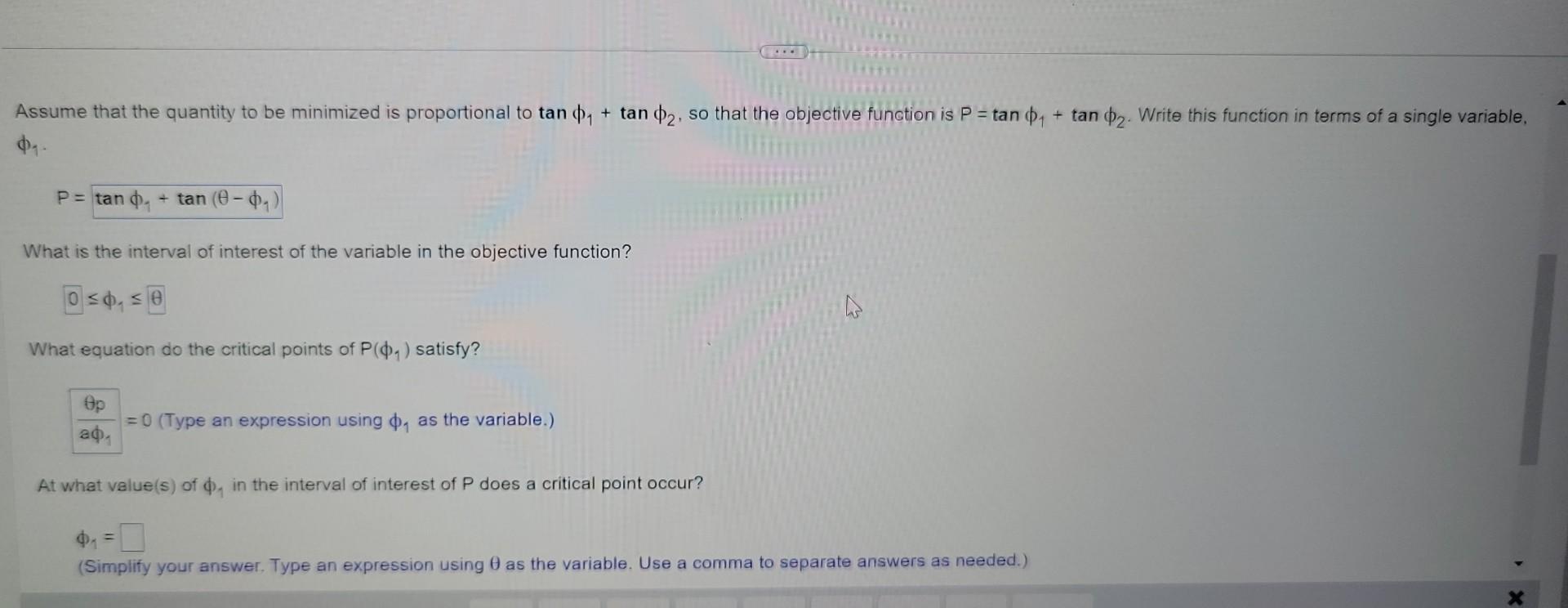Write this function in terms of a single variable ${?}_1$ $P=\tan {?}_1+\tan \left(??{?}_1\right)$ Assume that the quantity to be minimized is proportional to $\tan {?}_1+\tan {?}_2$, so that the objective function is $P=\tan {?}_1+\tan {?}_2$. Write this function in terms of a single variable, ${?}_1$ $P=\tan {?}_1+\tan \left(??{?}_1\right)$ What is the interval of interest of the variable in the objective function? $?{?}_1??$ What equation do the critical points of $P\left({?}_1\right)$ satisfy? $\frac{?p}{a{?}_1}=0$ (Type an expression using ${?}_1$ as the variable.) At what value(s) of ${?}_1$ in the interval of interest of $P$ does a critical point occur? ${?}_1=$ (Simplify your answer. Type an expression using $?$ as the variable. 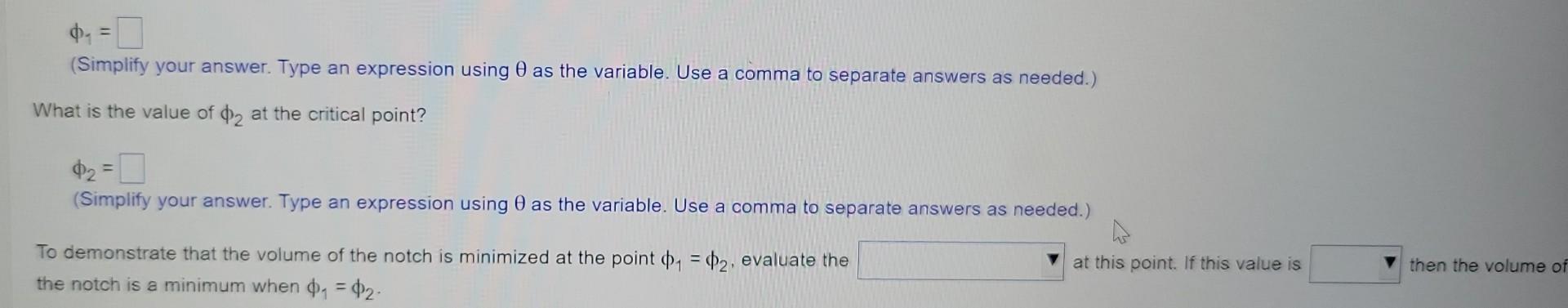Use a comma to separate answers as needed.) ${?}_1=$ (Simplify your answer. Type an expression using $?$ as the variable. Use a comma to separate answers as needed.) What is the value of ${?}_2$ at the critical point? ${?}_2=$ (Simplify your answer. Type an expression using $?$ as the variable. Use a comma to separate answers as needed.) To demonstrate that the volume of the notch is minimized at the point ${?}_1={?}_2$, evaluate the the notch is a minimum when ${?}_1={?}_2$. at this point. If this value is then the volume o