(Solved): A non-standard cell or half-cell potential can be calculated using the Nernst Equation: \[ E=E^{\c ...

A non-standard cell or half-cell potential can be calculated using the Nernst Equation: \[ E=E^{\circ}-\frac{R T}{n F} \ln Q \] where \( \mathrm{E}= \) potential under non-standard conditions \( \mathrm{E}^{\circ}= \) standard potential \( \mathrm{R}= \) ideal gas constant \( \mathrm{T}= \) kelvin temperature \( \mathbf{n}= \) number of moles of electrons for the reaction as written \( \mathbf{F}= \) charge carried by 1 mol of electrons \( \mathbf{Q}= \) reaction quotient It is customary to use the equation in a form where numerical values are substituted for \( \mathbf{R}, \mathbf{T} \) and \( \mathbf{F} \) at a temperature of \( 25^{\circ} \mathrm{C} \). For \[ \begin{aligned} \mathrm{R} & =8.314 \mathrm{~J} \mathrm{~mol}^{-1} \mathrm{~K}^{-1} \\ \mathrm{~T} & =298.15 \mathrm{~K} \\ \mathrm{~F} & =96.485 \mathrm{~J}^{-1} \mathrm{~mol}^{-1} \\ \mathrm{RT} & =\frac{\left(8.314 \mathrm{~J} \mathrm{~mol}^{-1} \mathrm{~K}^{-1}\right)(298.15 \mathrm{~K})}{\mathrm{F}}=0.485 \mathrm{~J} \mathrm{~V}^{-1} \mathrm{~mol}^{-1} \end{aligned} \] and the Nernst equation with the potentials in volts is: \[ \mathrm{E}=\mathrm{E}^{\circ}-\frac{0.0257}{\mathrm{n}} \ln \mathrm{Q} \quad \text { natural logarithm } \] Sometimes it is more convenient to use base-10 logarithms and the substitution of \( 2.303 \) log for In Is made. \( (2.303 \times 0.0257=0.0592) \) Then, the Nernst equation for base-10 logs at \( 25{ }^{\circ} \mathrm{C} \) is: \[ E=E^{\circ}-\frac{0.0592}{n} \log Q \] A common student error is to use the wrong kind of logarithm. Be sure, when you choose an equation, to use the correct logarithm. 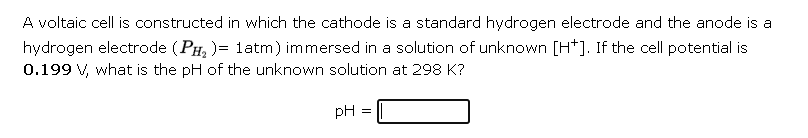A voltaic cell is constructed in which the cathode is a standard hydrogen electrode and the anode is a hydrogen electrode \( \left.\left(P_{H_{2}}\right)=1 \mathrm{~atm}\right) \) immersed in a solution of unknown \( \left[\mathrm{H}^{+}\right] \). If the cell potential is \( 0.199 \mathrm{~V} \), what is the \( \mathrm{pH} \) of the unknown solution at \( 298 \mathrm{~K} \) ? Consider the following cell reaction: \[ \mathrm{Mn}(\mathrm{s})+2 \mathrm{H}^{+}(? \mathrm{M}) \longrightarrow \mathrm{Mn}^{2+}(1.00 \mathrm{M})+\mathrm{H}_{2}(g)(1.00 \mathrm{~atm}) \] If the cell potential at \( 298 \mathrm{~K} \) is \( 0.368 \) volts, what is the \( \mathrm{pH} \) of the hydrogen electrode? \[ \mathrm{pH}= \]