(Solved): A moment, \( \mathrm{M}=8 \mathrm{kip}-\mathrm{ft} \) is applied on two ends of the beam as shown i ...

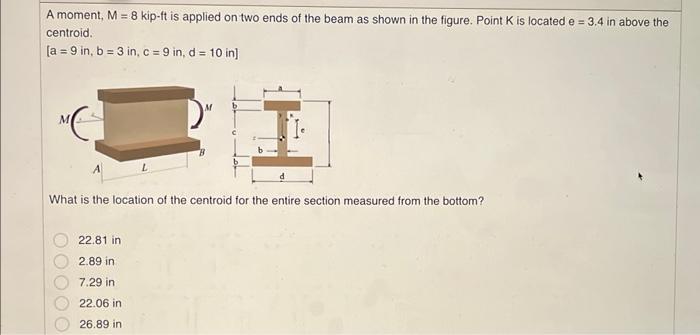
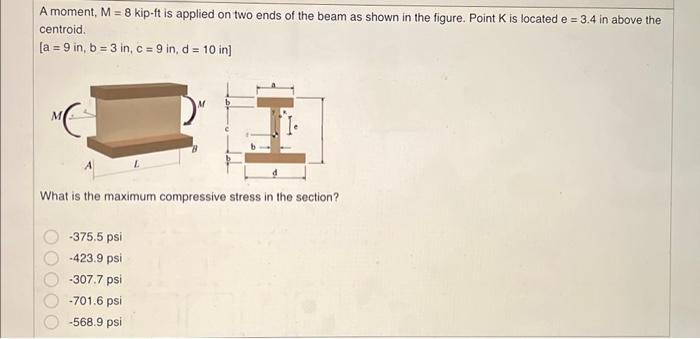
A moment, \( \mathrm{M}=8 \mathrm{kip}-\mathrm{ft} \) is applied on two ends of the beam as shown in the figure. Point \( \mathrm{K} \) is located \( \theta=3.4 \) in above the centroid. \[ [a=9 \text { in, } b=3 \text { in, } c=9 \text { in, } d=10 \text { in }] \] What is the location of the centroid for the entire section measured from the bottom? 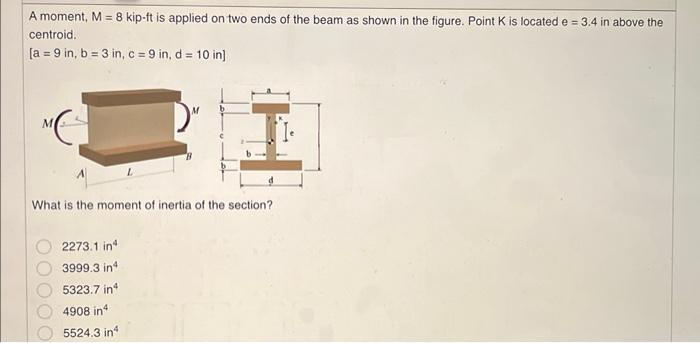\[ \begin{array}{l} 22.81 \text { in } \\ 2.89 \text { in } \\ 7.29 \text { in } \\ 22.06 \text { in } \\ 26.89 \text { in } \end{array} \] A moment, \( M=8 \) kip \( \cdot \mathrm{ft} \) is applied on two ends of the beam as shown in the figure. Point \( \mathrm{K} \) is located \( \mathrm{e}=3.4 \) in above the centroid. \[ \text { [ } a=9 \text { in, } b=3 \text { in, } c=9 \text { in, } d=10 \text { in ] } \] What is the moment of inertia of the section? 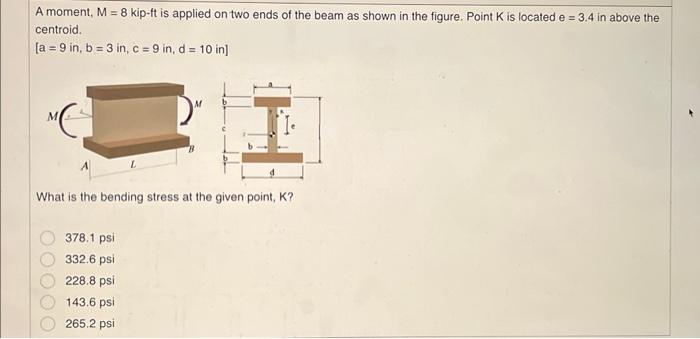\[ \begin{array}{l} 2273.1 \mathrm{in}^{4} \\ 3999.3 \mathrm{in}^{4} \\ 5323.7 \mathrm{in}^{4} \\ 4908 \mathrm{in}^{4} \\ 5524.3 \mathrm{in}^{4} \end{array} \] A moment, \( \mathrm{M}=8 \mathrm{kip} \cdot \mathrm{ft} \) is applied on two ends of the beam as shown in the figure. Point \( \mathrm{K} \) is located \( \theta=3.4 \) in above the centroid. \[ \text { [ } a=9 \text { in, } b=3 \text { in, } c=9 \text { in, } d=10 \text { in }] \] What is the bending stress at the given point, \( K \) ? \[ 378.1 \mathrm{psi} \] \[ \begin{array}{l} 332.6 \mathrm{psi} \\ 228.8 \mathrm{psi} \\ 143.6 \mathrm{psi} \\ 265.2 \mathrm{psi} \end{array} \] A moment, \( M=8 \) kip-ft is applied on two ends of the beam as shown in the figure. Point \( K \) is located \( e=3.4 \) in above the centroid. \[ \text { [ } \mathrm{a}=9 \text { in, } b=3 \text { in, } c=9 \text { in, } d=10 \text { in] } \] What is the section modulus for the top of the top of the section? 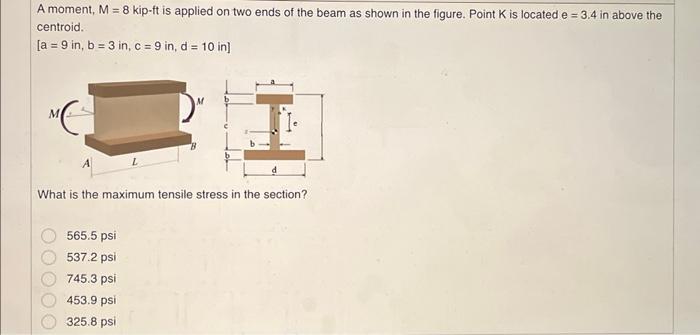\[ \begin{array}{l} 294.7 \mathrm{in}^{3} \\ 820.3 \mathrm{in}^{3} \\ 1071.4 \mathrm{in}^{3} \\ 730.2 \mathrm{in}^{3} \\ 1168.3 \mathrm{in}^{3} \end{array} \] A moment, \( M=8 \) kip-ft is applied on two ends of the beam as shown in the figure. Point \( \mathrm{K} \) is located \( e=3.4 \) in above the centroid. \[ \text { [ } a=9 \text { in, } b=3 \text { in, } c=9 \text { in, } d=10 \text { in }] \] What is the maximum tensile stress in the section? \[ \begin{array}{l} 565.5 \mathrm{psi} \\ 537.2 \mathrm{psi} \\ 745.3 \mathrm{psi} \\ 453.9 \mathrm{psi} \\ 325.8 \mathrm{psi} \end{array} \] A moment, \( M=8 \) kip-ft is applied on two ends of the beam as shown in the figure. Point \( K \) is located \( e=3.4 \) in above the centroid. \[ \text { [ } a=9 \text { in, } b=3 \text { in, } c=9 \text { in, } d=10 \text { in] } \] What is the maximum compressive stress in the section? \[ -375.5 \mathrm{psi} \] \[ \begin{array}{l} -423.9 \mathrm{psi} \\ -307.7 \mathrm{psi} \\ -701.6 \mathrm{psi} \\ -568.9 \mathrm{psi} \end{array} \]