(Solved): A linear, time-invariant system is described by the second-order differential ...

???????

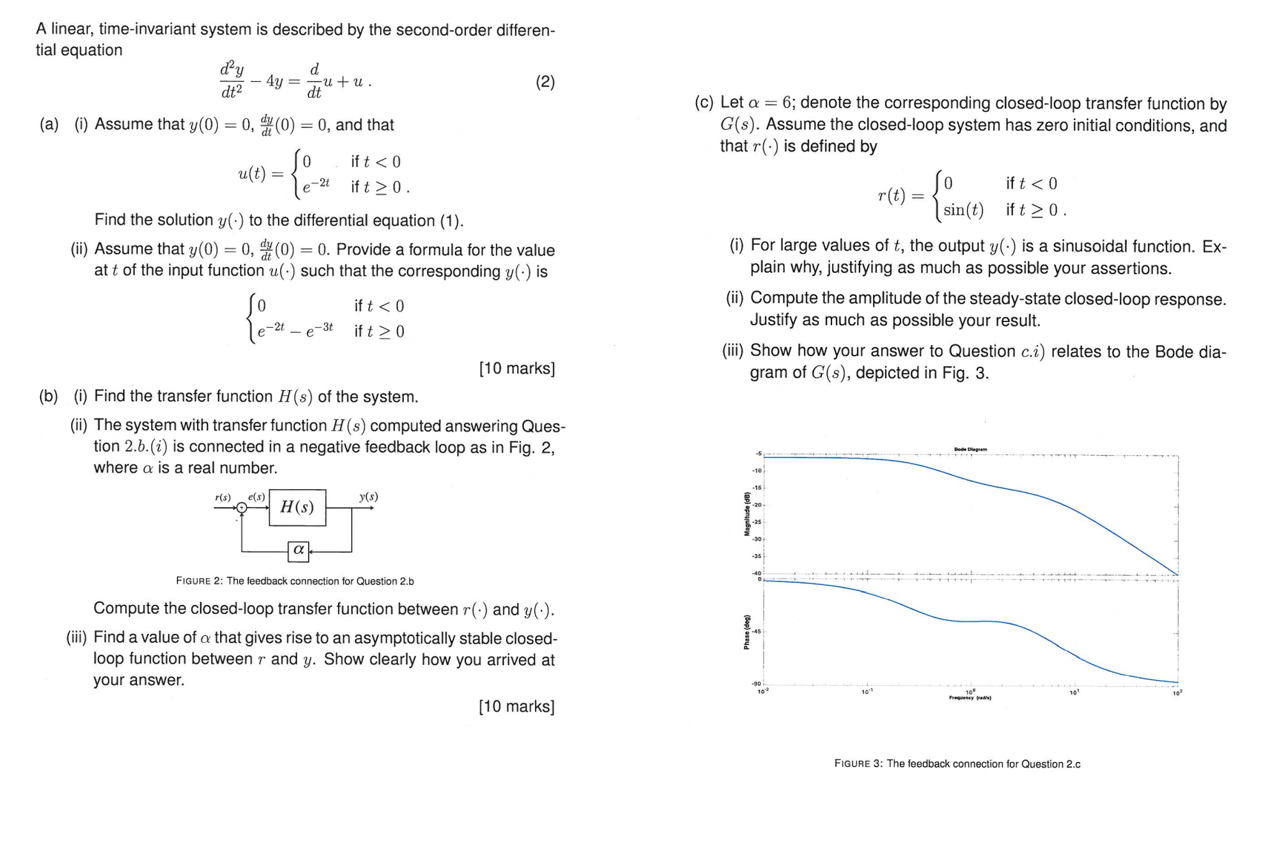
A linear, time-invariant system is described by the second-order differential equation \[ \frac{d^{2} y}{d t^{2}}-4 y=\frac{d}{d t} u+u . \] (c) Let \( \alpha=6 \); denote the corresponding closed-loop transfer function by (a) (i) Assume that \( y(0)=0, \frac{d y}{d t}(0)=0 \), and that \( G(s) \). Assume the closed-loop system has zero initial conditions, and \[ u(t)=\left\{\begin{array}{ll} 0 & \text { if } t<0 \\ e^{-2 t} & \text { if } t \geq 0 \end{array}\right. \] that \( r(\cdot) \) is defined by Find the solution \( y(\cdot) \) to the differential equation (1). \[ r(t)=\left\{\begin{array}{ll} 0 & \text { if } t<0 \\ \sin (t) & \text { if } t \geq 0 \end{array}\right. \] (ii) Assume that \( y(0)=0, \frac{d y}{d t}(0)=0 \). Provide a formula for the value (i) For large values of \( t \), the output \( y(\cdot) \) is a sinusoidal function. Exat \( t \) of the input function \( u(\cdot) \) such that the corresponding \( y(\cdot) \) is plain why, justifying as much as possible your assertions. \[ \left\{\begin{array}{ll} 0 & \text { if } t<0 \\ e^{-2 t}-e^{-3 t} & \text { if } t \geq 0 \end{array}\right. \] (ii) Compute the amplitude of the steady-state closed-loop response. Justify as much as possible your result. [10 marks] (iii) Show how your answer to Question \( c . i \) ) relates to the Bode diagram of \( G(s) \), depicted in Fig. 3. (b) (i) Find the transfer function \( H(s) \) of the system. (ii) The system with transfer function \( H(s) \) computed answering Question 2.b. (i) is connected in a negative feedback loop as in Fig. 2, where \( \alpha \) is a real number. FIGUAE 2: The feedback connection for Question 2.b Compute the closed-loop transfer function between \( r(\cdot) \) and \( y(\cdot) \). (iii) Find a value of \( \alpha \) that gives rise to an asymptotically stable closedloop function between \( r \) and \( y \). Show clearly how you arrived at your answer. [10 marks] FIGURE 3: The feedback connection for Question 2.c