(Solved): A generalization of the damped pendulum equation discussed in the text, or a damped spring-mass sys ...

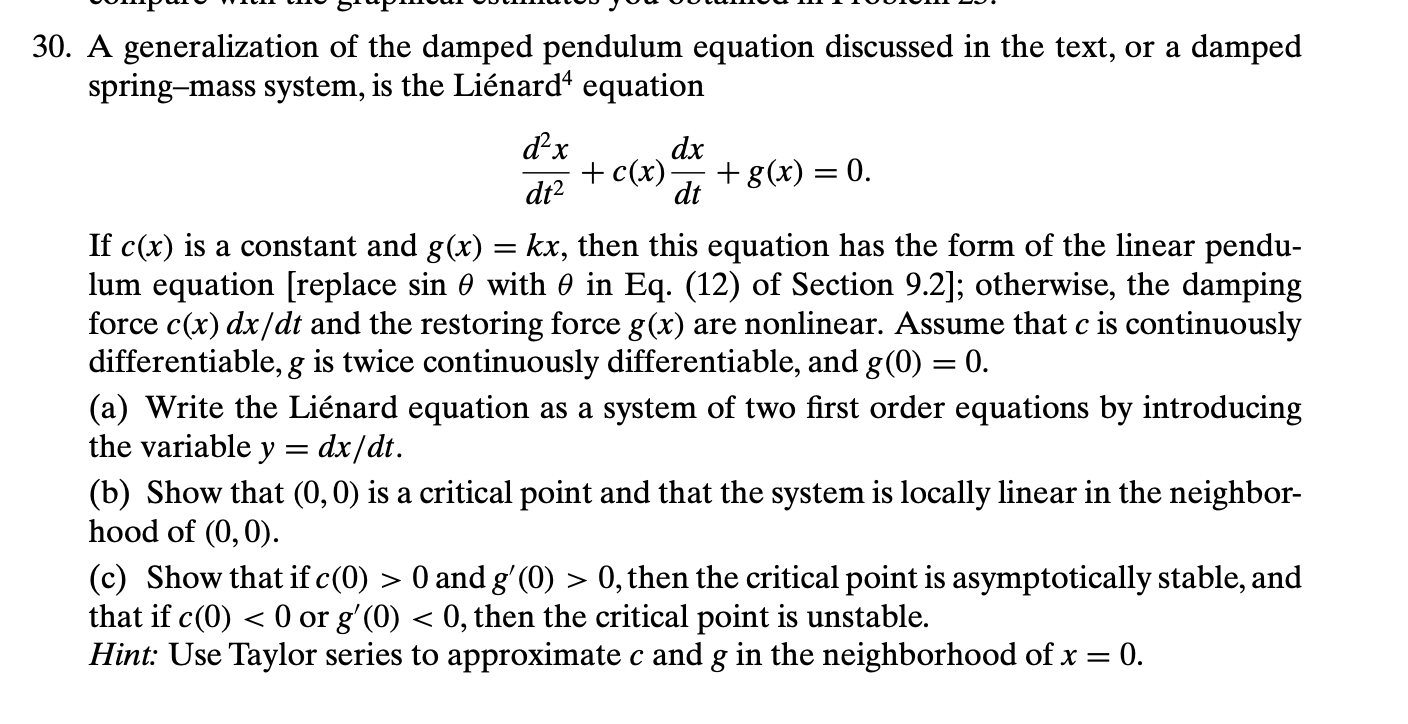
A generalization of the damped pendulum equation discussed in the text, or a damped spring-mass system, is the Liénard ${}^4$ equation $\frac{d^{2}x}{d{t}^2}+c(x)\frac{dx}{dt}+g(x)=0$ If $c(x)$ is a constant and $g(x)=kx$, then this equation has the form of the linear pendulum equation [replace $\sin ?$ with $?$ in Eq. (12) of Section 9.2]; otherwise, the damping force $c(x)dx/dt$ and the restoring force $g(x)$ are nonlinear. Assume that $c$ is continuously differentiable, $g$ is twice continuously differentiable, and $g(0)=0$. (a) Write the Liénard equation as a system of two first order equations by introducing the variable $y=dx/dt$. (b) Show that $(0,0)$ is a critical point and that the system is locally linear in the neighborhood of $(0,0)$. (c) Show that if $c(0)>0$ and $g^{?}(0)>0$, then the critical point is asymptotically stable, and that if $c(0)<0$ or $g^{?}(0)<0$, then the critical point is unstable. Hint: Use Taylor series to approximate $c$ and $g$ in the neighborhood of $x=0$.