(Solved): A circular loop of wire can be used as a radio antenna. If an \( 21.6-c m \)-diameter antenna is l ...

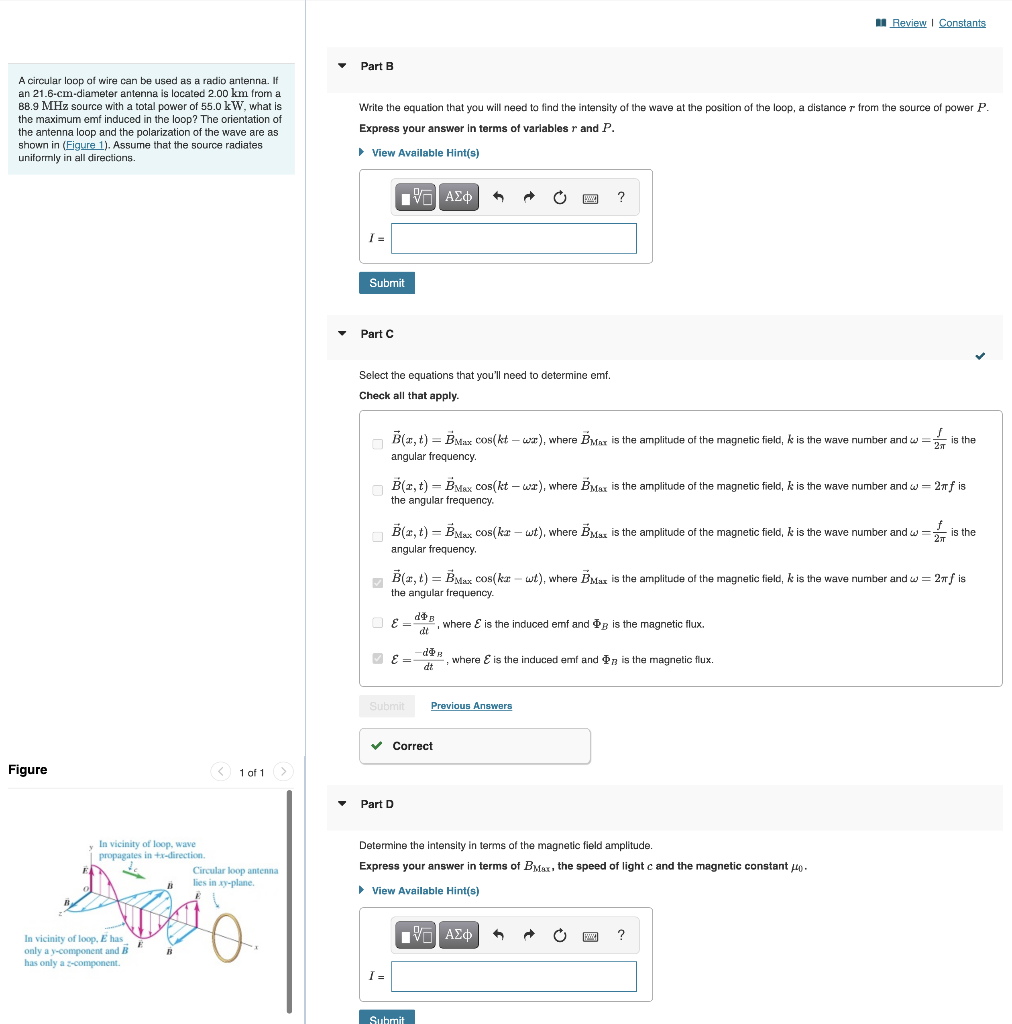
A circular loop of wire can be used as a radio antenna. If an \( 21.6-c m \)-diameter antenna is located \( 2.00 \mathrm{~km} \) from a BB.9 MHz source with a total power of \( 55.0 \mathrm{~kW} \), what is Write the equation that you will need to find the intensity of the wave at the position of the loop, a distance \( r \) from the source of power \( P \). the maximum emf induced in the loop? The orientation of Express your answer in terms of variables \( r \) and \( P \). the antenna loop and the polarization of the wave are as shown in (Figure 1). Assume that the source radiates uniformly in all directions. Part C Select the equations that you'll need to determine emf. Check all that apply. \( \vec{B}(x, t)=\vec{B}_{\text {Max }} \cos (k t-\omega x) \), where \( \vec{B}_{\text {Max }} \) is the amplitude of the magnetic field, \( k \) is the wave number and \( \omega=\frac{f}{2 \pi} \) is the angular frequency. \( \vec{B}(x, t)=\vec{B}_{\mathrm{Max}} \cos (k t-\omega x) \), where \( \vec{B}_{\mathrm{Max}} \) is the amplitude of the magnetic field, \( k \) is the wave number and \( \omega=2 \pi f \) is the angular frequency. \( \vec{B}(x, t)=\vec{B}_{\text {Max }} \cos (k x-\omega t) \), where \( \vec{B}_{\mathrm{Biax}} \) is the amplitude of the magnetic field, \( k \) is the wave number and \( \omega=\frac{f}{2 \pi} \) is the angular frequency. \( \vec{B}(x, t)=\vec{B}_{\text {Max }} \cos (k x-\omega t) \), where \( \vec{B}_{\mathrm{B} a x} \) is the amplitude of the magnetic field, \( k \) is the wave number and \( \omega=2 \pi f \) is the angular frequency. \( \mathcal{E}=\frac{d \Phi_{B}}{d t} \), where \( \mathcal{E} \) is the induced emf and \( \Phi_{B} \) is the magnetic flux. \( \mathcal{E}=\frac{-d \Phi_{R}}{d t} \), where \( \mathcal{E} \) is the induced emf and \( \Phi_{B} \) is the magnetic flux. Figure 1 of 1 Part D Determine the intensity in terms of the magnetic field amplitude. Express your answer in terms of \( B_{\mathrm{Max}} \), the speed of light \( c \) and the magnetic constant \( \mu_{0} \).