(Solved): A capacitor with spherical geometry as in Figure 1 forms an inner conductive sphere with radius a ...

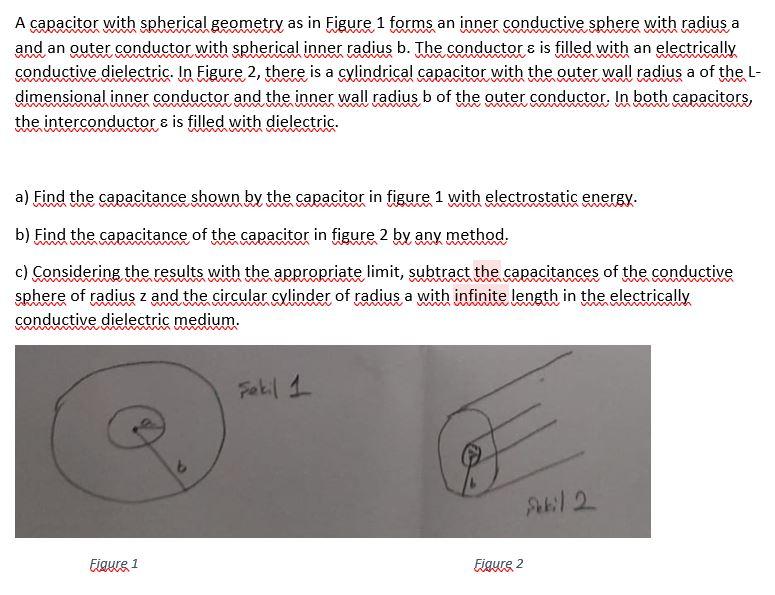
A capacitor with spherical geometry as in Figure 1 forms an inner conductive sphere with radius a and an outer conductor with spherical inner radius b. The conductor \( \varepsilon \) is filled with an electrically conductive dielectric. In Figure 2, there is a cylindrical capacitor with the outer wall radius a of the Ldimensional inner conductor and the inner wall radius \( b \) of the outer conductor. In both capacitors, the interconductor \( \varepsilon \) is filled with dielectric. a) Find the capacitance shown by the capacitor in figure 1 with electrostatic energy. b) Find the capacitance of the capacitor in figure 2 by any method. c) Considering the results with the appropriate limit, subtract the capacitances of the conductive sphere of radius \( z \) and the circular cylinder of radius a with infinite length in the electrically conductive dielectric medium. Eiqure 1 Eigure 2