(Solved): A beam of uniform rectangular cross section is to be cut from a \( \log \) having a circular cross ...

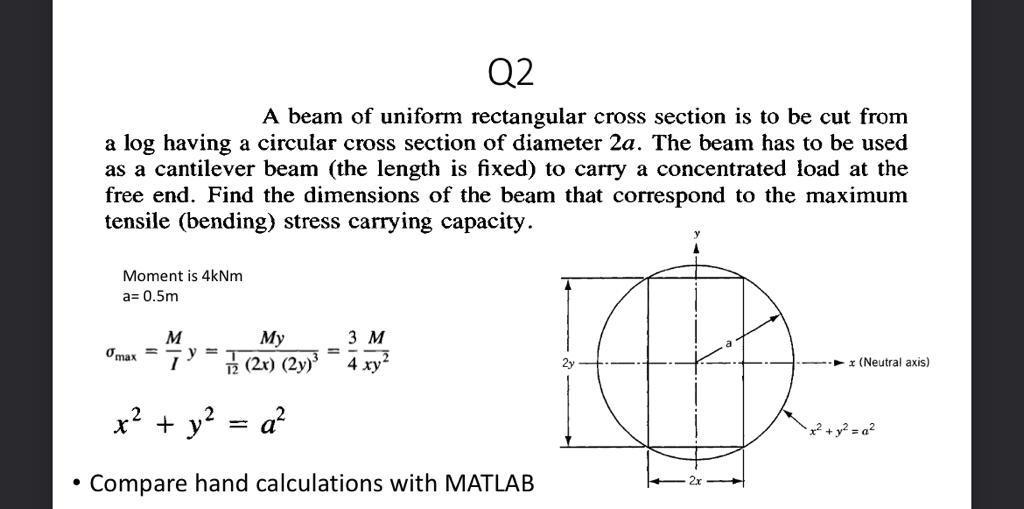
A beam of uniform rectangular cross section is to be cut from a \( \log \) having a circular cross section of diameter \( 2 a \). The beam has to be used as a cantilever beam (the length is fixed) to carry a concentrated load at the free end. Find the dimensions of the beam that correspond to the maximum tensile (bending) stress carrying capacity. \[ \begin{array}{l} \text { Moment is } 4 \mathrm{kNm} \\ a=0.5 \mathrm{~m} \\ \sigma_{\max }=\frac{M}{I} y=\frac{M y}{\frac{1}{12}(2 x)(2 y)^{3}}=\frac{3}{4} \frac{M}{x y^{2}} \\ x^{2}+y^{2}=a^{2} \end{array} \] - Compare hand calculations with MATLAB