(Solved): A batch reactor experiment on algal uptake of nutrients from solution is expected to follow mixed ...

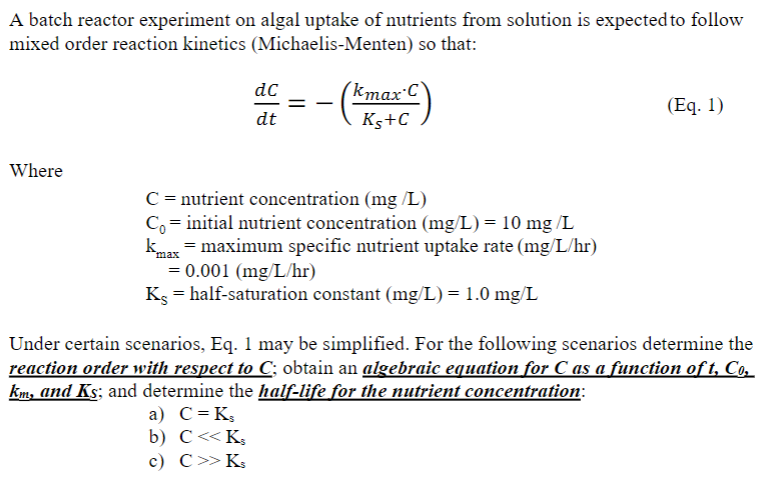
A batch reactor experiment on algal uptake of nutrients from solution is expected to follow mixed order reaction kinetics (Michaelis-Menten) so that: \[ \frac{d C}{d t}=-\left(\frac{k_{\max } \cdot C}{K_{s}+C}\right) \] Where \[ \begin{array}{l} \mathrm{C}=\text { nutrient concentration ( } \mathrm{mg} / \mathrm{L}) \\ \mathrm{C}_{0}=\text { initial nutrient concentration }(\mathrm{mg} / \mathrm{L})=10 \mathrm{mg} / \mathrm{L} \\ \mathrm{k}_{\max }=\text { maximum specific nutrient uptake rate }(\mathrm{mg} / \mathrm{L} / \mathrm{hr}) \\ \quad=0.001(\mathrm{mg} / \mathrm{L} / \mathrm{hr}) \\ \mathrm{K}_{\mathrm{S}}=\text { half-saturation constant }(\mathrm{mg} / \mathrm{L})=1.0 \mathrm{mg} / \mathrm{L} \end{array} \] Under certain scenarios, Eq. 1 may be simplified. For the following scenarios determine the reaction order with respect to \( C \); obtain an algebraic equation for \( C \) as a function of \( t, C_{0} \), \( \underline{k}_{m} \), and \( K_{s} \); and determine the half-life for the nutrient concentration: a) \( \mathrm{C}=\mathrm{K}_{\mathrm{s}} \) b) \( \mathrm{C}<\mathrm{K}_{3} \) c) \( \mathrm{C} \gg \mathrm{K}_{s} \)