a) Applying the momentum conservation principle, prove that fluid velocity variation in the radial direction for fully developed laminar flow in a horizontal pipe is given by the following relation: [ u(r)=- frac{R^{2}}{4 mu} left( frac{d P}{d x} right) left(1- frac{r^{2}}{R^{2}} right) ] where ( mathrm{x} ) is distance along fl solutionspile.com

Question

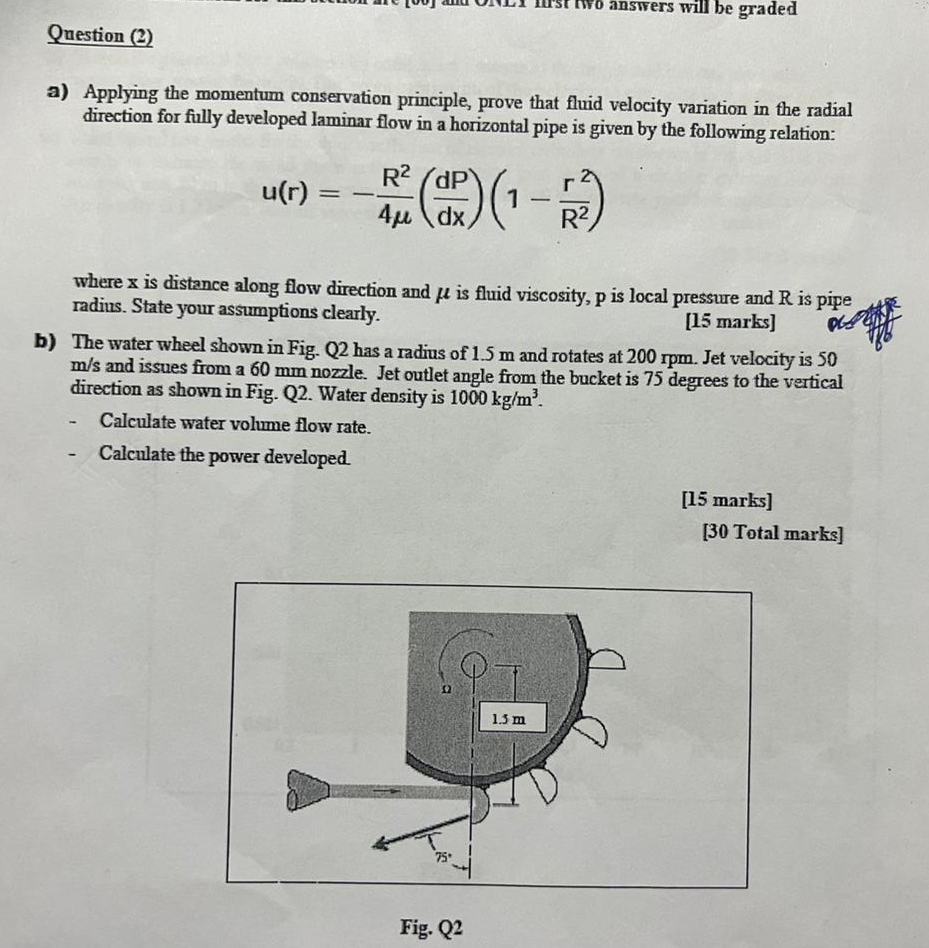
a) Applying the momentum conservation principle, prove that fluid velocity variation in the radial direction for fully developed laminar flow in a horizontal pipe is given by the following relation:   [ u(r)=-  frac{R^{2}}{4   mu}  left(  frac{d P}{d x}  right)  left(1-  frac{r^{2}}{R^{2}}  right)   ] where   (   mathrm{x}   ) is distance along flow direction and   (   mu   ) is fluid viscosity,   (   mathrm{p}   ) is local pressure and   (   mathrm{R}   ) is pipe radius. State your assumptions clearly. [15 marks] b) The water wheel shown in Fig. Q2 has a radius of   ( 1.5   mathrm{~m}   ) and rotates at   ( 200   mathrm{rpm}   ). Jet velocity is 50   (   mathrm{m} /   mathrm{s}   ) and issues from a   ( 60   mathrm{~mm}   ) nozzle. Jet outlet angle from the bucket is 75 degrees to the vertical direction as shown in Fig. Q2. Water density is   ( 1000   mathrm{~kg} /   mathrm{m}^{3}   ). - Calculate water volume flow rate. - Calculate the power developed. [15 marks] [30 Total marks] Fig. Q2 solutionspile.com

Accepted Answer

Expert Answer to -  a) Applying the momentum conservation principle, prove that fluid velocity variation in the radial

Answer

Solution for -  a) Applying the momentum conservation principle, prove that fluid velocity variation in the radial

Answer

This an additional answer to -  a) Applying the momentum conservation principle, prove that fluid velocity variation in the radial

(Solved): a) Applying the momentum conservation principle, prove that fluid velocity variation in the radial ...

View Expert Answer

Expert Answer

Buy This Answer $5

Place Order

We Provide Services Across The Globe