(Solved): A and B What is that figure's "punchline?" (Referring to panel A of the figure in question 1.) (For ...

 

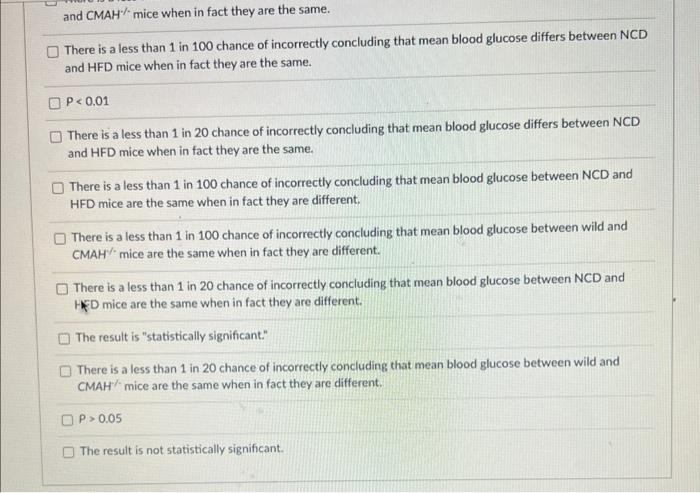
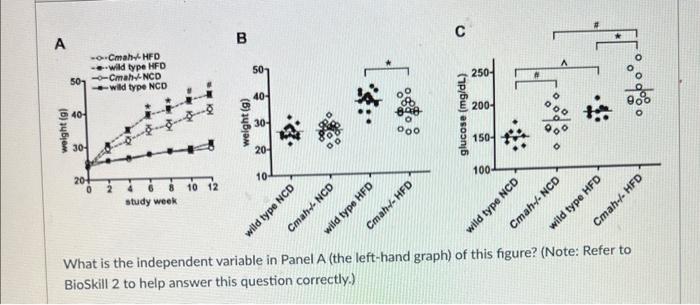
What is that figure's "punchline?" (Referring to panel A of the figure in question 1.) (For an example of what this means, see Figure B2.1, panel (c) of the textbook. But note: you are answering the question about the diabetes study in Box 2, panel A of the figure, not the graph in the textbook.) Write your response in the space below. Compare blood glucose concentrations of wild type mice fed HFD to \( \mathrm{Cmah}^{-/-} \)mice fed HFD. Find the bracket connecting those 2 groups in the figure, and note that there is a single asterisk above the bracket. What exactly does that asterisk mean? Mark all that apply. See BioSkill 3 in the textbook, especially Table B3.1 and the "Check your understanding" box. There is a less than 1 in 100 chance of incorrectly concluding that mean blood glucose differs between wild and \( \mathrm{CMAH}^{-/} \)mice when in fact they are the same. \( p>0.01 \) \( P<0.05 \) There is a less than 1 in 20 chance of incorrectly concluding that mean blood glucose differs between wild and \( \mathrm{CMAH}^{-/} \)mice when in fact they are the same. There is a less than 1 in 100 chance of incorrectly concluding that mean blood glucose differs between NCD and HFD mice when in fact they are the same. \( P<0.01 \) There is a less than 1 in 20 chance of incorrectly concluding that mean blood glucose differs between NCD and HFD mice when in fact they are the same. There is a less than 1 in 100 chance of incorrectly concluding that mean blood glucose between NCD and HFD mice are the same when in fact they are different. There is a less than 1 in 100 chance of incorrectly concluding that mean blood glucose between wild and \( \mathrm{CMAH}^{\prime /} \) mice are the same when in fact they are different. There is a less than 1 in 20 chance of incorrectly concluding that mean blood glucose between NCD and HFD mice are the same when in fact they are different. The result is "statistically significant." There is a less than 1 in 20 chance of incorrectly concluding that mean blood glucose between wild and \( \mathrm{CMAH}^{\prime} \) mice are the same when in fact they are different. and \( \mathrm{CMAH}^{\%} \) mice when in fact they are the same. There is a less than 1 in 100 chance of incorrectly concluding that mean blood glucose differs between NCD and HFD mice when in fact they are the same. \[ p<0.01 \] There is a less than 1 in 20 chance of incorrectly concluding that mean blood glucose differs between NCD and HFD mice when in fact they are the same. There is a less than 1 in 100 chance of incorrectly concluding that mean blood glucose between NCD and HFD mice are the same when in fact they are different. There is a less than 1 in 100 chance of incorrectly concluding that mean blood glucose between wild and \( \mathrm{CMAH}^{/ /} \)mice are the same when in fact they are different. There is a less than 1 in 20 chance of incorrectly concluding that mean blood glucose between NCD and HED mice are the same when in fact they are different. The result is "statistically significant." There is a less than 1 in 20 chance of incorrectly concluding that mean blood glucose between wild and \( \mathrm{CMAH}^{\prime} \) mice are the same when in fact they are different. \[ P>0.05 \] The result is not statistically significant. What is the independent variable in Panel A (the left-hand graph) of this figure? (Note: Refer to BioSkill 2 to help answer this question correctly.)