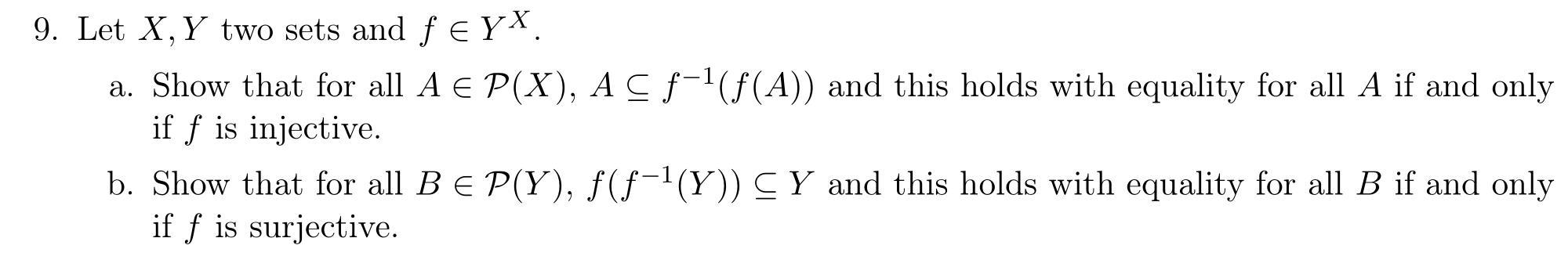(Solved): 9. Let \( X, Y \) two sets and \( f \in Y^{X} \). a. Show that for all \( A \in \mathcal{P}(X), A ...

9. Let \( X, Y \) two sets and \( f \in Y^{X} \). a. Show that for all \( A \in \mathcal{P}(X), A \subseteq f^{-1}(f(A)) \) and this holds with equality for all \( A \) if and only if \( f \) is injective. b. Show that for all \( B \in \mathcal{P}(Y), f\left(f^{-1}(Y)\right) \subseteq Y \) and this holds with equality for all \( B \) if and only if \( f \) is surjective.