Home /
Expert Answers /
Other Math /
8-let-t-mathbb-r-4-rightarrow-mathbb-r-3-be-the-linear-transformation-defined-by-pa933
(Solved): 8. Let \( T: \mathbb{R}^{4} \rightarrow \mathbb{R}^{3} \) be the linear transformation defined by ...
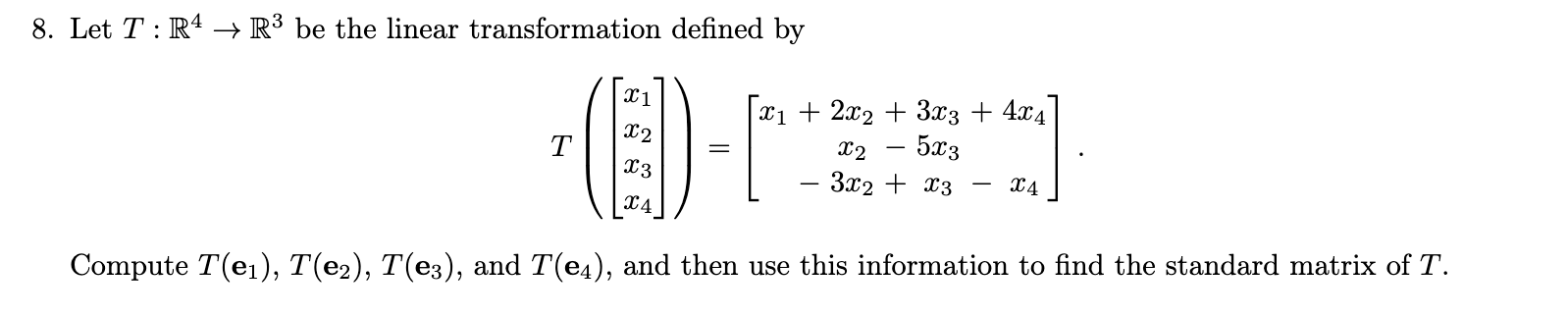
8. Let \( T: \mathbb{R}^{4} \rightarrow \mathbb{R}^{3} \) be the linear transformation defined by \[ T\left(\left[\begin{array}{l} x_{1} \\ x_{2} \\ x_{3} \\ x_{4} \end{array}\right]\right)=\left[\begin{array}{c} x_{1}+2 x_{2}+3 x_{3}+4 x_{4} \\ x_{2}-5 x_{3} \\ -3 x_{2}+x_{3}-x_{4} \end{array}\right] . \] Compute \( T\left(\mathbf{e}_{1}\right), T\left(\mathbf{e}_{2}\right), T\left(\mathbf{e}_{3}\right) \), and \( T\left(\mathbf{e}_{4}\right) \), and then use this information to find the standard matrix of \( T \).
Expert Answer
The Linear Transformation T: R4 ?R3 is defined by T([x1x2x3x4])=[x1+2x2+3x3+4x4x2?5x3?3x2+x3?x4] and e1=([1000]), e2=([0100]), e3=([0010]), e4=([0001]