(Solved): 8. Atoms in a solid vibrate about their respective equilibrium positions with small amplitudes. De ...

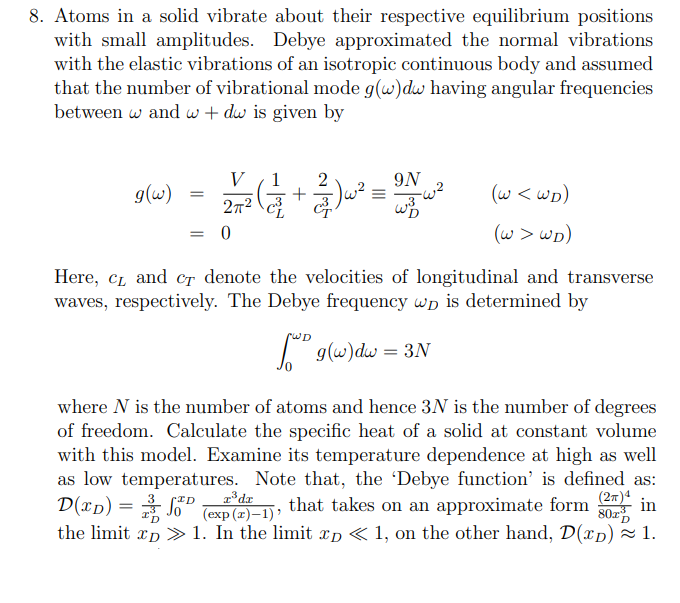
8. Atoms in a solid vibrate about their respective equilibrium positions with small amplitudes. Debye approximated the normal vibrations with the elastic vibrations of an isotropic continuous body and assumed that the number of vibrational mode \( g(\omega) d \omega \) having angular frequencies between \( \omega \) and \( \omega+d \omega \) is given by \[ \begin{aligned} g(\omega) & =\frac{V}{2 \pi^{2}}\left(\frac{1}{c_{L}^{3}}+\frac{2}{c_{T}^{3}}\right) \omega^{2} \equiv \frac{9 N}{\omega_{D}^{3}} \omega^{2} & & \left(\omega<\omega_{D}\right) \\ & =0 & & \left(\omega>\omega_{D}\right) \end{aligned} \] Here, \( c_{L} \) and \( c_{T} \) denote the velocities of longitudinal and transverse waves, respectively. The Debye frequency \( \omega_{D} \) is determined by \[ \int_{0}^{\omega_{D}} g(\omega) d \omega=3 N \] where \( N \) is the number of atoms and hence \( 3 N \) is the number of degrees of freedom. Calculate the specific heat of a solid at constant volume with this model. Examine its temperature dependence at high as well as low temperatures. Note that, the 'Debye function' is defined as: \( \mathcal{D}\left(x_{D}\right)=\frac{3}{x_{D}^{3}} \int_{0}^{x_{D}} \frac{x^{3} d x}{(\exp (x)-1)} \), that takes on an approximate form \( \frac{(2 \pi)^{4}}{80 x_{D}^{3}} \) in the limit \( x_{D} \gg 1 \). In the limit \( x_{D} \ll 1 \), on the other hand, \( \mathcal{D}\left(x_{D}\right) \approx 1 \).