(Solved): 7. For the high-pass filter circuit shown below, (a) Derive \( \mathrm{H}(\mathrm{j} \omega)=v_{o} ...

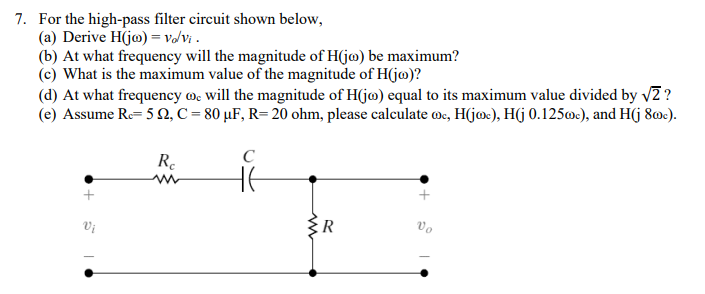
7. For the high-pass filter circuit shown below, (a) Derive \( \mathrm{H}(\mathrm{j} \omega)=v_{o} / v_{i} \). (b) At what frequency will the magnitude of \( \mathrm{H}(\mathrm{j} \omega) \) be maximum? (c) What is the maximum value of the magnitude of \( \mathrm{H}(\mathrm{j} \omega) \) ? (d) At what frequency \( \omega_{\mathrm{c}} \) will the magnitude of \( \mathrm{H}(\mathrm{j} \omega) \) equal to its maximum value divided by \( \sqrt{2} \) ? (e) Assume \( \mathrm{R}_{\mathrm{c}}=5 \Omega, \mathrm{C}=80 \mu \mathrm{F}, \mathrm{R}=20 \mathrm{ohm} \), please calculate \( \omega_{\mathrm{c}}, \mathrm{H}\left(\mathrm{j} \omega_{\mathrm{c}}\right), \mathrm{H}\left(\mathrm{j} 0.125 \omega_{c}\right) \), and \( \mathrm{H}\left(\mathrm{j} 8 \omega_{c}\right) \).