(Solved): 6) (Bessel functions) The Bessel differential equation is given by \[ x^{2} y^{\prime \prime}+x y^ ...

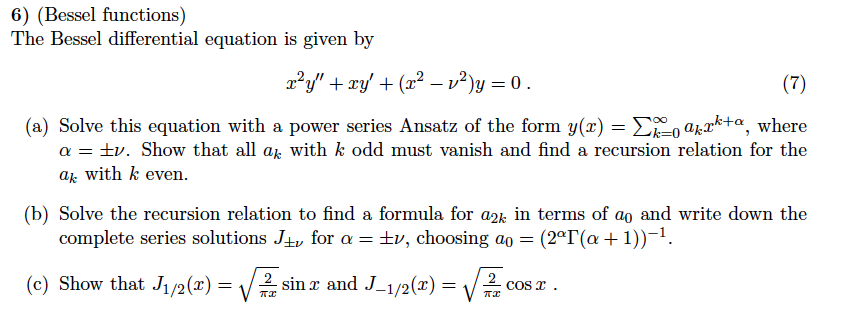
6) (Bessel functions) The Bessel differential equation is given by \[ x^{2} y^{\prime \prime}+x y^{\prime}+\left(x^{2}-\nu^{2}\right) y=0 . \] (a) Solve this equation with a power series Ansatz of the form \( y(x)=\sum_{k=0}^{\infty} a_{k} x^{k+\alpha} \), where \( \alpha=\pm \nu \). Show that all \( a_{k} \) with \( k \) odd must vanish and find a recursion relation for the \( a_{k} \) with \( k \) even. (b) Solve the recursion relation to find a formula for \( a_{2 k} \) in terms of \( a_{0} \) and write down the complete series solutions \( J_{\pm \nu} \) for \( \alpha=\pm \nu \), choosing \( a_{0}=\left(2^{\alpha} \Gamma(\alpha+1)\right)^{-1} \). (c) Show that \( J_{1 / 2}(x)=\sqrt{\frac{2}{\pi x}} \sin x \) and \( J_{-1 / 2}(x)=\sqrt{\frac{2}{\pi x}} \cos x \).