(Solved): 5. \( \phi: \mathbb{Z} / 2 \mathbb{Z} \longrightarrow \operatorname{Aut}(\mathbb{Z}) \) is defined ...

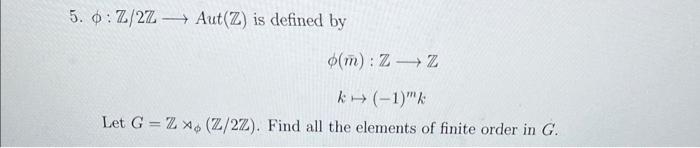
5. \( \phi: \mathbb{Z} / 2 \mathbb{Z} \longrightarrow \operatorname{Aut}(\mathbb{Z}) \) is defined by \[ \begin{array}{c} \phi(\bar{m}): \mathbb{Z} \longrightarrow \mathbb{Z} \\ k \mapsto(-1)^{m} k \end{array} \] Let \( G=\mathbb{Z} \rtimes_{\phi}(\mathbb{Z} / 2 \mathbb{Z}) \). Find all the elements of finite order in \( G \).