Home /
Expert Answers /
Advanced Math /
5-linear-independence-and-spanning-suppose-v-has-a-basis-beta-mathbf-u-mathbf-v-pa986
(Solved): #5 Linear Independence and Spanning Suppose \( V \) has a basis \( \beta=\{\mathbf{u}, \mathbf{v}, ...
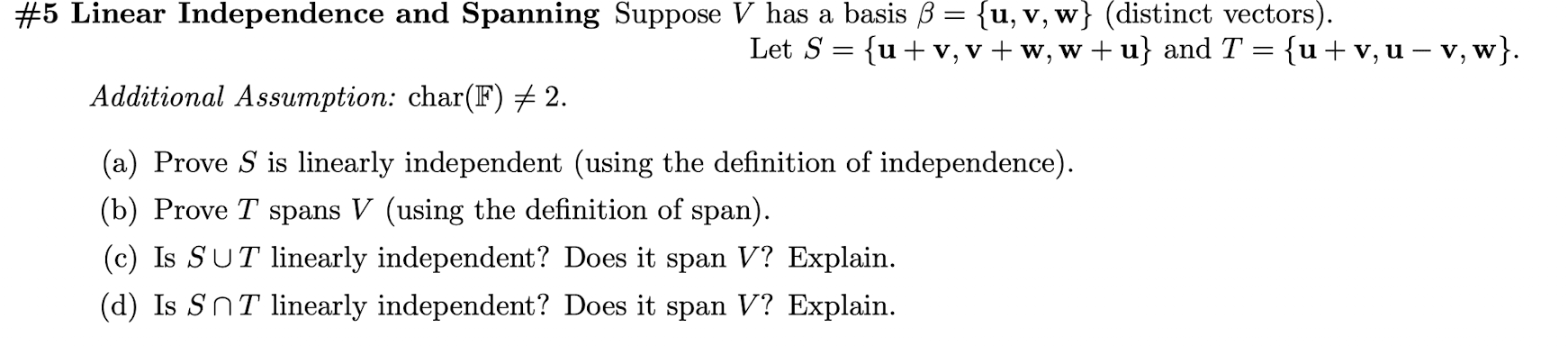
#5 Linear Independence and Spanning Suppose \( V \) has a basis \( \beta=\{\mathbf{u}, \mathbf{v}, \mathbf{w}\} \) (distinct vectors). Let \( S=\{\mathbf{u}+\mathbf{v}, \mathbf{v}+\mathbf{w}, \mathbf{w}+\mathbf{u}\} \) and \( T=\{\mathbf{u}+\mathbf{v}, \mathbf{u}-\mathbf{v}, \mathbf{w}\} \). Additional Assumption: \( \operatorname{char}(\mathbb{F}) \neq 2 \). (a) Prove \( S \) is linearly independent (using the definition of independence). (b) Prove \( T \) spans \( V \) (using the definition of span). (c) Is \( S \cup T \) linearly independent? Does it span \( V \) ? Explain. (d) Is \( S \cap T \) linearly independent? Does it span \( V \) ? Explain.
Expert Answer
Let V be a vector space and ?={u,v,w} Be basis for V. This implies, dim?(V)=3. Let, S={u+v,v+w,w+u}T={u+v,u?v,w}