(Solved): 5. Let \( \mathbf{F}(\mathbf{R})=\{f \mid f: \mathbf{R} \rightarrow \mathbf{R}\} \) be the vector ...

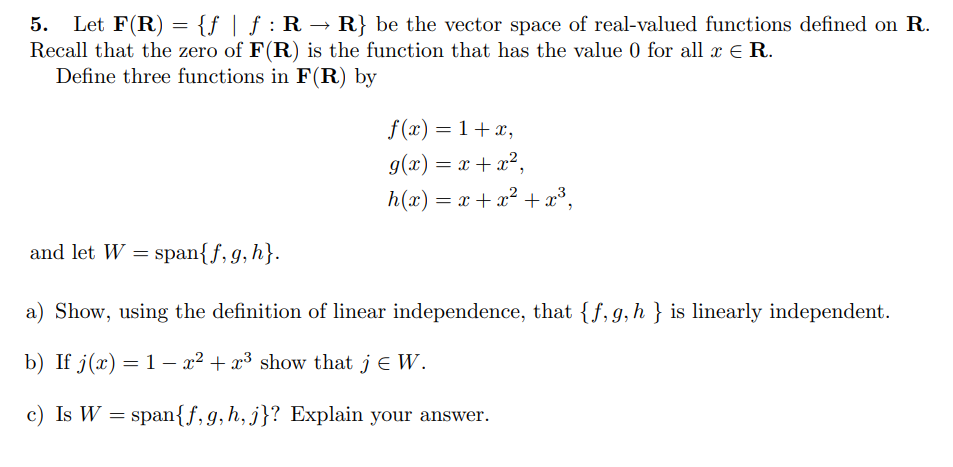
5. Let \( \mathbf{F}(\mathbf{R})=\{f \mid f: \mathbf{R} \rightarrow \mathbf{R}\} \) be the vector space of real-valued functions defined on \( \mathbf{R} \). Recall that the zero of \( \mathbf{F}(\mathbf{R}) \) is the function that has the value 0 for all \( x \in \mathbf{R} \). Define three functions in \( \mathbf{F}(\mathbf{R}) \) by \[ \begin{array}{l} f(x)=1+x \\ g(x)=x+x^{2}, \\ h(x)=x+x^{2}+x^{3}, \end{array} \] and let \( W=\operatorname{span}\{f, g, h\} \). a) Show, using the definition of linear independence, that \( \{f, g, h\} \) is linearly independent. b) If \( j(x)=1-x^{2}+x^{3} \) show that \( j \in W \). c) Is \( W=\operatorname{span}\{f, g, h, j\} ? \) Explain your answer.