(Solved): 4. Consider the regression through the origin model \( Y_{i}=\beta_{1} X_{i}+ ...

???????

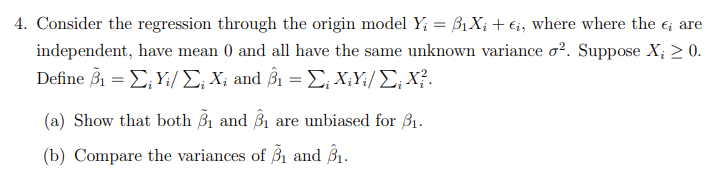
4. Consider the regression through the origin model \( Y_{i}=\beta_{1} X_{i}+\epsilon_{i} \), where where the \( \epsilon_{i} \) are independent, have mean 0 and all have the same unknown variance \( \sigma^{2} \). Suppose \( X_{i} \geq 0 \). Define \( \tilde{\beta}_{1}=\sum_{i} Y_{i} / \sum_{i} X_{i} \) and \( \hat{\beta}_{1}=\sum_{i} X_{i} Y_{i} / \sum_{i} X_{i}^{2} \) (a) Show that both \( \tilde{\beta}_{1} \) and \( \hat{\beta}_{1} \) are unbiased for \( \beta_{1} \). (b) Compare the variances of \( \tilde{\beta}_{1} \) and \( \hat{\beta}_{1} \).