(Solved): 4. Consider a simple harmonic oscillator. Use the orthogonality and the recurrence relations for t ...

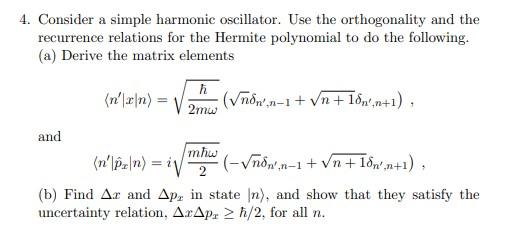
4. Consider a simple harmonic oscillator. Use the orthogonality and the recurrence relations for the Hermite polynomial to do the following. (a) Derive the matrix elements \[ \left\langle n^{\prime}|x| n\right\rangle=\sqrt{\frac{\hbar}{2 m \omega}}\left(\sqrt{n} \delta_{n^{\prime}, n-1}+\sqrt{n+1} \delta_{n^{\prime}, n+1}\right), \] and \[ \left\langle n^{\prime}\left|\hat{p}_{x}\right| n\right\rangle=i \sqrt{\frac{m \hbar \omega}{2}}\left(-\sqrt{n} \delta_{n^{\prime}, n-1}+\sqrt{n+1} \delta_{n^{\prime}, n+1}\right), \] (b) Find \( \Delta x \) and \( \Delta p_{x} \) in state \( |n\rangle \), and show that they satisfy the uncertainty relation, \( \Delta x \Delta p_{x} \geq \hbar / 2 \), for all \( n \).