(Solved): 4. A spin \\( 1 / 2 \\) particle is placed in a magnetic field and interacts through the Hamiltonia ...

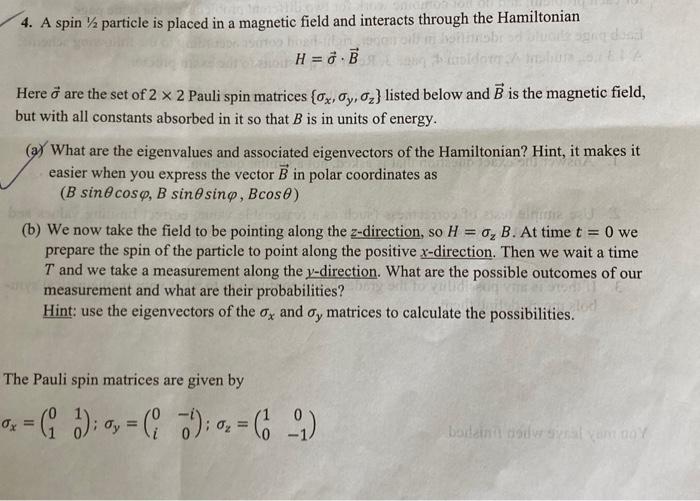
4. A spin \\( 1 / 2 \\) particle is placed in a magnetic field and interacts through the Hamiltonian \\[ H=\\vec{\\sigma} \\cdot \\vec{B} \\] Here \\( \\vec{\\sigma} \\) are the set of \\( 2 \\times 2 \\) Pauli spin matrices \\( \\left\\{\\sigma_{x}, \\sigma_{y}, \\sigma_{z}\\right\\} \\) listed below and \\( \\vec{B} \\) is the magnetic field, but with all constants absorbed in it so that \\( B \\) is in units of energy. (a) What are the eigenvalues and associated eigenvectors of the Hamiltonian? Hint, it makes it easier when you express the vector \\( \\vec{B} \\) in polar coordinates as \\[ (B \\sin \\theta \\cos \\varphi, B \\sin \\theta \\sin \\varphi, B \\cos \\theta) \\] (b) We now take the field to be pointing along the \\( z \\)-direction, so \\( H=\\sigma_{z} B \\). At time \\( t=0 \\) we prepare the spin of the particle to point along the positive \\( x \\)-direction. Then we wait a time \\( T \\) and we take a measurement along the \\( y \\)-direction. What are the possible outcomes of our measurement and what are their probabilities? Hint: use the eigenvectors of the \\( \\sigma_{x} \\) and \\( \\sigma_{y} \\) matrices to calculate the possibilities. The Pauli spin matrices are given by \\[ \\sigma_{x}=\\left(\\begin{array}{ll} 0 & 1 \\\\ 1 & 0 \\end{array}\\right) ; \\sigma_{y}=\\left(\\begin{array}{cc} 0 & -i \\\\ i & 0 \\end{array}\\right) ; \\sigma_{z}=\\left(\\begin{array}{cc} 1 & 0 \\\\ 0 & -1 \\end{array}\\right) \\]