(Solved): "Right-hand-rule" for the Cartesian unit vectors - Make the following shape with your RIGHT hand: - ...

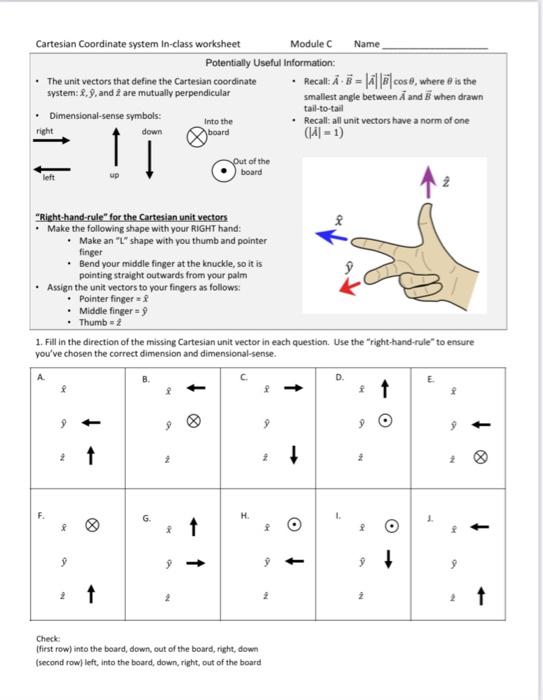
"Right-hand-rule" for the Cartesian unit vectors - Make the following shape with your RIGHT hand: - Make an "L" shape with you thumb and pointer finger - Bend your middle finger at the knuckle, so it is pointing straight outwards from your palm - Assign the unit vectors to your fingers as follows: - Pointer finger $$=\hat{x}$$ - Middle finger $$=y$$ - Thumb $$=\hat{2}$$ 1. Fial in the direction of the missing Cartesian unit vector in each question. Use the "right-hand-rule" to ensure you've chosen the correct dimension and dimensional-sense. check: (first row) into the board, down, out of the board, right, down (second row) left, into the board, down, right, out of the board 2. Fill in the missing blanks in the following exercises, which find the dot product between any two Cartesian unit. vectors. $$\begin{array}{l}\hat{x}?\hat{y}=?\hat{x}??\hat{y}?\cos (?)\hat{x}?\hat{z}=?\hat{x}??\hat{z}?\cos (?)\hat{z}?\hat{y}=?\hat{2}??\hat{y}?\cos (?)\\ =())()\cos ()=())()\cos ()=())()\cos ()\\ =\mathrm{m}=\end{array}$$ 3. The vector below is a 2-dimensional vector. It "lives in the plane of the board". Therefore, it is automatically perpendicular to any vector that points perfectly "into the board" or "out of the board". Use the vector and the chosen coordinate system to perform the following exercises. Chosen coordinate system Redraw $$\vec{r}$$ tail to tail with each of the Cartesian unit vectors. Then write an expression (or an exact value) for the smallest angle that $$\vec{r}$$ makes with each unit vector, in terms of the given angle $$?$$. 4. The vector below is a 2-dimensional vector. it "lives in the plane of the board". Therefore, it is automatically perpendicular to any vector that points perfectly "into the board" or "out of the board". Use the vector and the chosen coordinate system to perform the following exercises. Redraw $$\vec{F}$$ tail to tail with each of the Cartesian unit vectors. Then write an expression (or an exact value) for the smallest angle that $$\vec{F}$$ makes with each unit vector, in terms of the given angle $$?$$.