(Solved): 3. The horizontal cylinder of soil as shown in Figure below. Assume \( \mathrm{L}=10 \mathrm{~cm} \ ...

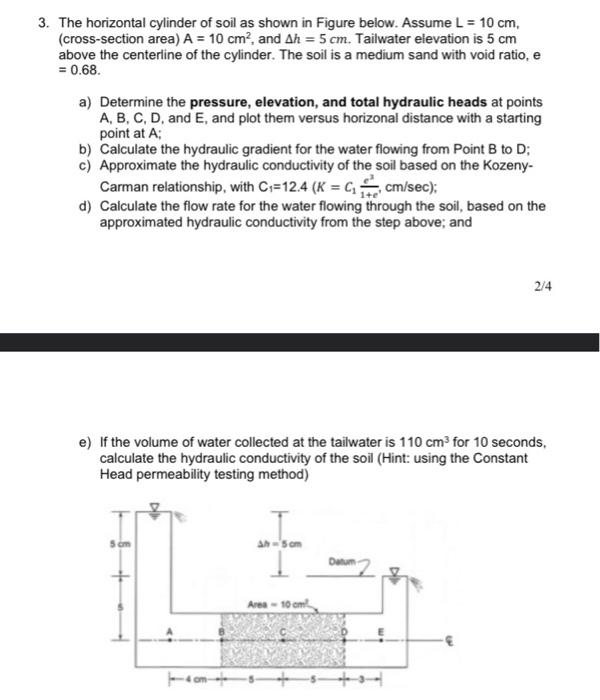
3. The horizontal cylinder of soil as shown in Figure below. Assume \( \mathrm{L}=10 \mathrm{~cm} \), (cross-section area) \( \mathrm{A}=10 \mathrm{~cm}^{2} \), and \( \Delta h=5 \mathrm{~cm} \). Tailwater elevation is \( 5 \mathrm{~cm} \) above the centerline of the cylinder. The soil is a medium sand with void ratio, \( e \) \( =0.68 \) a) Determine the pressure, elevation, and total hydraulic heads at points A, B, C, D, and \( E \), and plot them versus horizonal distance with a starting point at \( \mathrm{A} \); b) Calculate the hydraulic gradient for the water flowing from Point B to D; c) Approximate the hydraulic conductivity of the soil based on the KozenyCarman relationship, with \( \mathrm{C}_{1}=12.4\left(K=C_{1} \frac{e^{3}}{1+e}, \mathrm{~cm} / \mathrm{sec}\right) \); d) Calculate the flow rate for the water flowing through the soil, based on the approximated hydraulic conductivity from the step above; and e) If the volume of water collected at the tailwater is \( 110 \mathrm{~cm}^{3} \) for 10 seconds, calculate the hydraulic conductivity of the soil (Hint: using the Constant Head permeability testing method)