Home /
Expert Answers /
Advanced Math /
3-show-that-y-sin-x-and-y-cos-x-are-linearly-independent-functions-but-that-their-wron-pa534
(Solved): 3. Show that y, = sin x and y = cos x are linearly independent functions, but that their Wron ...
3. Show that y, = sin x² and y? = cos x² are linearly independent functions, but that their Wronskian vanishes at x=0. Why does this imply that there is no differential equation of the form y" + p(x)y'+q(x)y=0, with both p and q continuous everywhere, having both y, and Y? as solutions? Two functions defined on an open interval I are said to be linearly independent on I provided that (1) Y? The functions y, = sin x² and y? = cos x² are linearly independent because Y2 function and = (3) Y1 (Type expressions using x as the variable.) Given two functions f and g, the Wronskian off and g is the determinant defined as follows. W(f.g) = (4) The Wronskian for these functions, W(sinx, cos x²)=[ (Type an expression using x as the variable.) (4) (1) the two functions are not identical on 1. neither is a constant multiple of the other. Why does this imply that there is no differential equation of the form y+p(xly + g(x)y= 0, with both p and q continuous everywhere, having both y, and y2 as solutions? OA Such an equation would require that the Wronskian is nonzero for every point on the real line Since W (sin x² cos x)=0 for x=0, such an equation cannot exist. LOG 00 OB. Such an equation would require that the Wronskian is nonzero for at most one point on the real line. Since W (sin x², cos x²) #0 for any x #0, such an equation cannot exist. = (5). 9 C. Such an equation would require that the Wronskian is constant-valued. Since w (sin x², cos x²) = 0 for x = 0 and W (sin x². cos x²) #0 for x0, such an equation cannot exist D. Such an equation would require that the Wronskian is zero for every point on the real line. Since W (sin x² cos x²) #0 for x * 0, such an equation cannot exist f 9 neither can be expressed as the other raised to a constant exponent. neither is the sum of the other and a constant 9 9 fg Y2 fa 0000 H a constant-valued function (5) fg-fg (6) sin lg-f'g fg-1g € (2) vanishes at x = 0 because it has a factor of (6). CO (2) is not ISS a constant-valued (3) is nol B
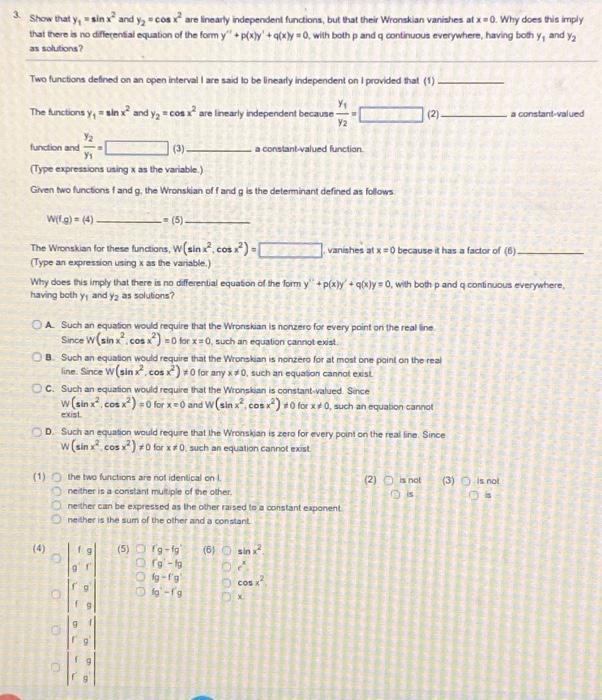
3. Show that and are linearty independent functions, but that their Wronskian vanishes at . Why does this imply that there is no diflecential equation of the form , with both and contimuous everywhere, having both and as solubons? Two functions defined on an open interval I are said to be linearly independent on I provided that (1) The functions and are finearly independent becatuse (2) a constant-valued function and (3) a constant-valued function. (Type exprestions using as the variable) Given two functions and , the Wronskian of and is the determinant defined as follows The Wronskian for these functions, (Type an exprestion using as the variable.) Why does this imply that there is no differential equaton of the form , with both and continuous everywhere, having both and as solubions? A. Such an equation would require that the Wronskian is nonzero for every point on the real fine Since for , such an equation cannot exist. B. Such an equation would require that the Wronskan is nonzero for at most one point on the real line. Since for any , such an equalon cannot exist. C. Such an equation would require that the Wronskan is constant valued. Sence for and for , such an equation cannot exist. D. Such an squation would requre that the Wronskan is zero for every point on the reak ine. Since far , such an equation cannot exist (1) the two functions are not identical on 1 . (2) is not (3) (1) is not neither is a constant mutiple of the ocher, is nether can be expressed as the other raised to a constant eaponent. nether is the sum of the other and a constant. (4) (5) (6)