(Solved): 3. A shock sensor has a high input impedance and produces a small voltage signal and is modeled by ...

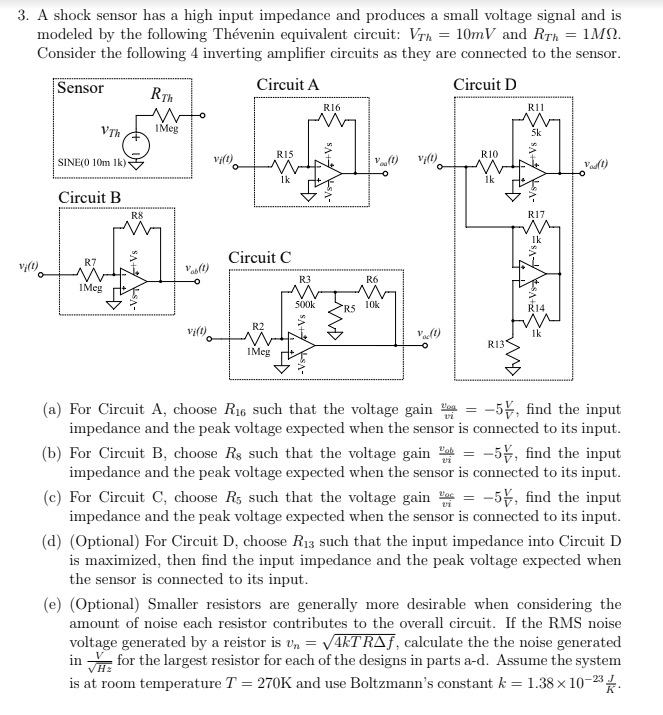
3. A shock sensor has a high input impedance and produces a small voltage signal and is modeled by the following Thévenin equivalent circuit: \( V_{T h}=10 \mathrm{mV} \) and \( R_{T h}=1 M \Omega \). Consider the following 4 inverting amplifier circuits as they are connected to the sensor. Circuit R (a) For Circuit \( \mathrm{A} \), choose \( R_{16} \) such that the voltage gain \( \frac{v_{a n}}{v i}=-5 \frac{V}{V} \), find the input impedance and the peak voltage expected when the sensor is connected to its input. (b) For Circuit B, choose \( R_{8} \) such that the voltage gain \( \frac{v_{o b}}{v i}=-5 \frac{V}{V} \), find the input impedance and the peak voltage expected when the sensor is connected to its input. (c) For Circuit C, choose \( R_{5} \) such that the voltage gain \( \frac{v_{a c}}{v i}=-5 \frac{V}{V} \), find the input impedance and the peak voltage expected when the sensor is connected to its input. (d) (Optional) For Circuit D, choose \( R_{13} \) such that the input impedance into Circuit D is maximized, then find the input impedance and the peak voltage expected when the sensor is connected to its input. (e) (Optional) Smaller resistors are generally more desirable when considering the amount of noise each resistor contributes to the overall circuit. If the RMS noise voltage generated by a reistor is \( v_{n}=\sqrt{4 k T R \Delta f} \), calculate the the noise generated in \( \frac{V}{\sqrt{H z}} \) for the largest resistor for each of the designs in parts a-d. Assume the system is at room temperature \( T=270 \mathrm{~K} \) and use Boltzmann's constant \( k=1.38 \times 10^{-23} \frac{\mathrm{J}}{\mathrm{K}} \).