(Solved): 3.4. In an aneurysm, a blood vessel becomes locally dilated. The cause of this dilation is unknown ...

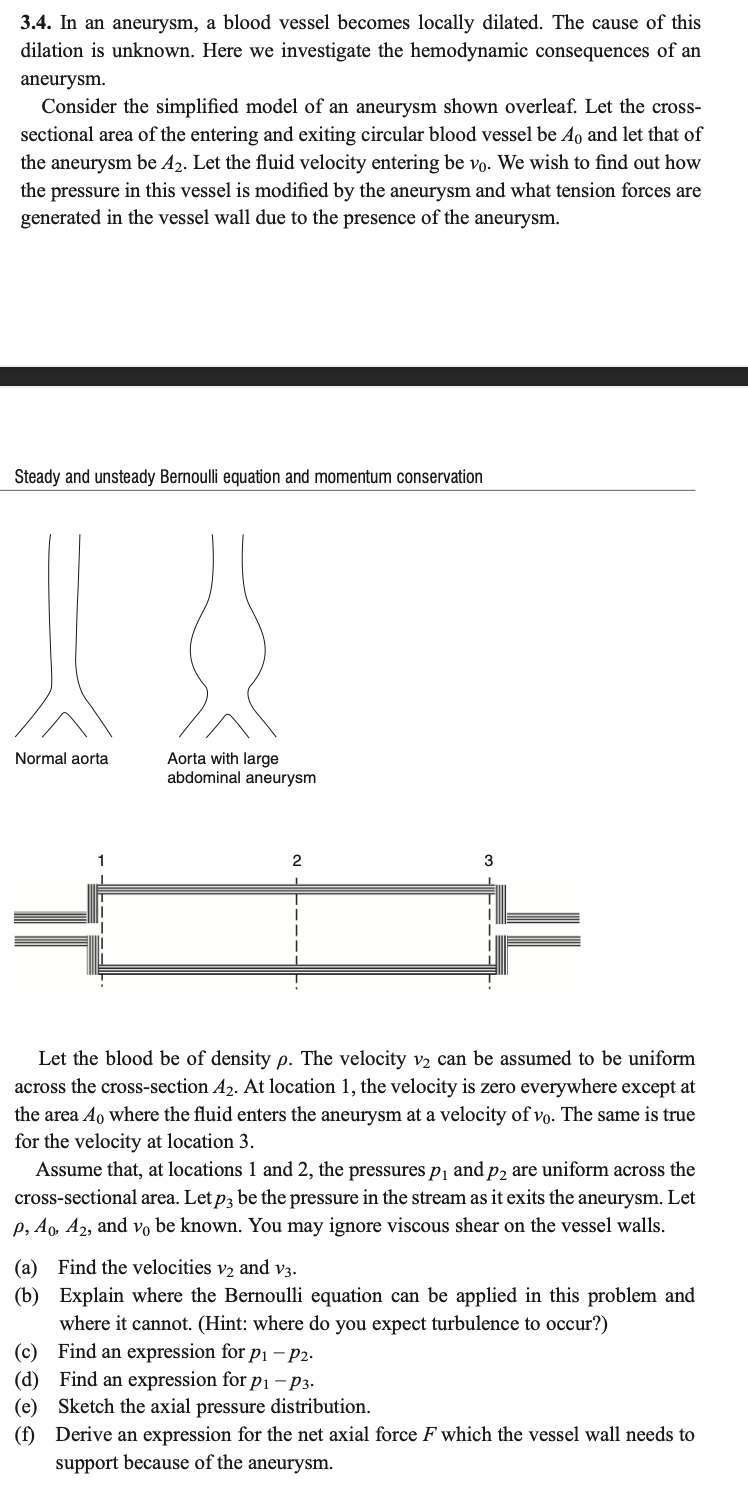
3.4. In an aneurysm, a blood vessel becomes locally dilated. The cause of this dilation is unknown. Here we investigate the hemodynamic consequences of an aneurysm. Consider the simplified model of an aneurysm shown overleaf. Let the crosssectional area of the entering and exiting circular blood vessel be \( A_{0} \) and let that of the aneurysm be \( A_{2} \). Let the fluid velocity entering be \( v_{0} \). We wish to find out how the pressure in this vessel is modified by the aneurysm and what tension forces are generated in the vessel wall due to the presence of the aneurysm. Steady and unsteady Bernoulli equation and momentum conservation Let the blood be of density \( \rho \). The velocity \( v_{2} \) can be assumed to be uniform across the cross-section \( A_{2} \). At location 1, the velocity is zero everywhere except at the area \( A_{0} \) where the fluid enters the aneurysm at a velocity of \( v_{0} \). The same is true for the velocity at location 3 . Assume that, at locations 1 and 2, the pressures \( p_{1} \) and \( p_{2} \) are uniform across the cross-sectional area. Let \( p_{3} \) be the pressure in the stream as it exits the aneurysm. Let \( \rho, A_{0}, A_{2} \), and \( v_{0} \) be known. You may ignore viscous shear on the vessel walls. (a) Find the velocities \( v_{2} \) and \( v_{3} \). (b) Explain where the Bernoulli equation can be applied in this problem and where it cannot. (Hint: where do you expect turbulence to occur?) (c) Find an expression for \( p_{1}-p_{2} \). (d) Find an expression for \( p_{1}-p_{3} \). (e) Sketch the axial pressure distribution. (f) Derive an expression for the net axial force \( F \) which the vessel wall needs to support because of the aneurysm.