(Solved): 2. Testing the "thin separation" assumption. Reconsider the pressure-driven flow problem betwee ...

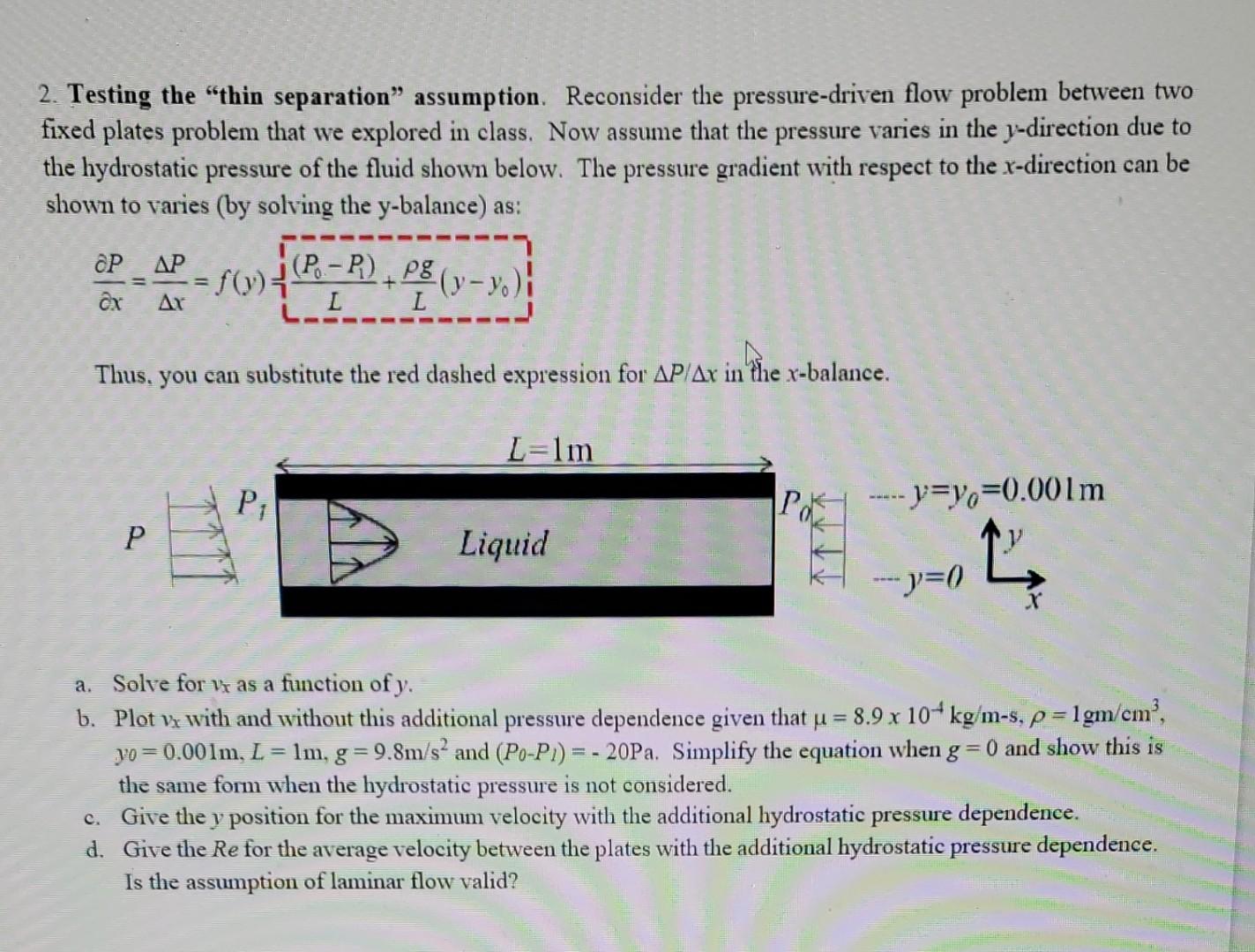
2. Testing the "thin separation" assumption. Reconsider the pressure-driven flow problem between two fixed plates problem that we explored in class. Now assume that the pressure varies in the $y$-direction due to the hydrostatic pressure of the fluid shown below. The pressure gradient with respect to the $x$-direction can be shown to varies (by solving the $y$-balance) as: $\frac{?P}{?x}=\frac{?P}{?x}=f(y)\frac{\left(P_0?P_1\right)}{L}+\frac{?g}{L}\left(y?y_0\right)!$ Thus, you can substitute the red dashed expression for $?P/?x$ in the $x$-balance. a. Solve for $v_x$ as a function of $y$. b. Plot $v_x$ with and without this additional pressure dependence given that $?=8.9\times 10^{?4}\mathrm{kg}/\mathrm{m}?\mathrm{s},?=1\mathrm{gm}/{\mathrm{cm}}^3$, $y_0=0.001\mathrm{m},L=1\mathrm{m},g=9.8\mathrm{m}/{\mathrm{s}}^2$ and $\left(P_{0?}{P}_l\right)=?20\mathrm{Pa}$. Simplify the equation when $g=0$ and show this is the same form when the hydrostatic pressure is not considered. c. Give the $y$ position for the maximum velocity with the additional hydrostatic pressure dependence. d. Give the $Re$ for the average velocity between the plates with the additional hydrostatic pressure dependence. Is the assumption of laminar flow valid?