(Solved): 2. In class, we showed that the Taylor series for \( e^{x} \) is \[ e^{x}=\sum_{n=0}^{\infty} \fra ...

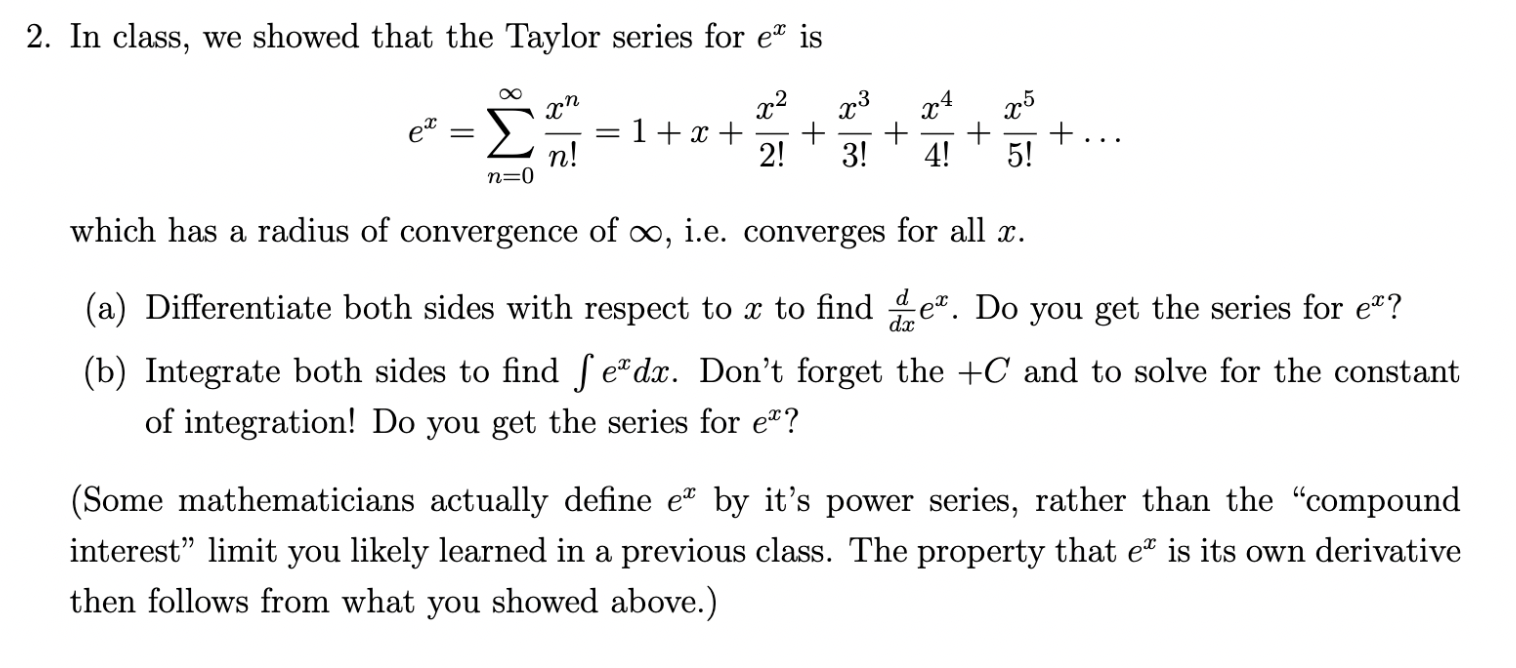
2. In class, we showed that the Taylor series for \( e^{x} \) is \[ e^{x}=\sum_{n=0}^{\infty} \frac{x^{n}}{n !}=1+x+\frac{x^{2}}{2 !}+\frac{x^{3}}{3 !}+\frac{x^{4}}{4 !}+\frac{x^{5}}{5 !}+\ldots \] which has a radius of convergence of \( \infty \), i.e. converges for all \( x \). (a) Differentiate both sides with respect to \( x \) to find \( \frac{d}{d x} e^{x} \). Do you get the series for \( e^{x} \) ? (b) Integrate both sides to find \( \int e^{x} d x \). Don't forget the \( +C \) and to solve for the constant of integration! Do you get the series for \( e^{x} ? \) (Some mathematicians actually define \( e^{x} \) by it's power series, rather than the "compound interest" limit you likely learned in a previous class. The property that \( e^{x} \) is its own derivative then follows from what you showed above.)