(Solved): 2.) Differential entropy, Chain rule, Polarization a) Assume the butterfly structure of a 22 DFT ...

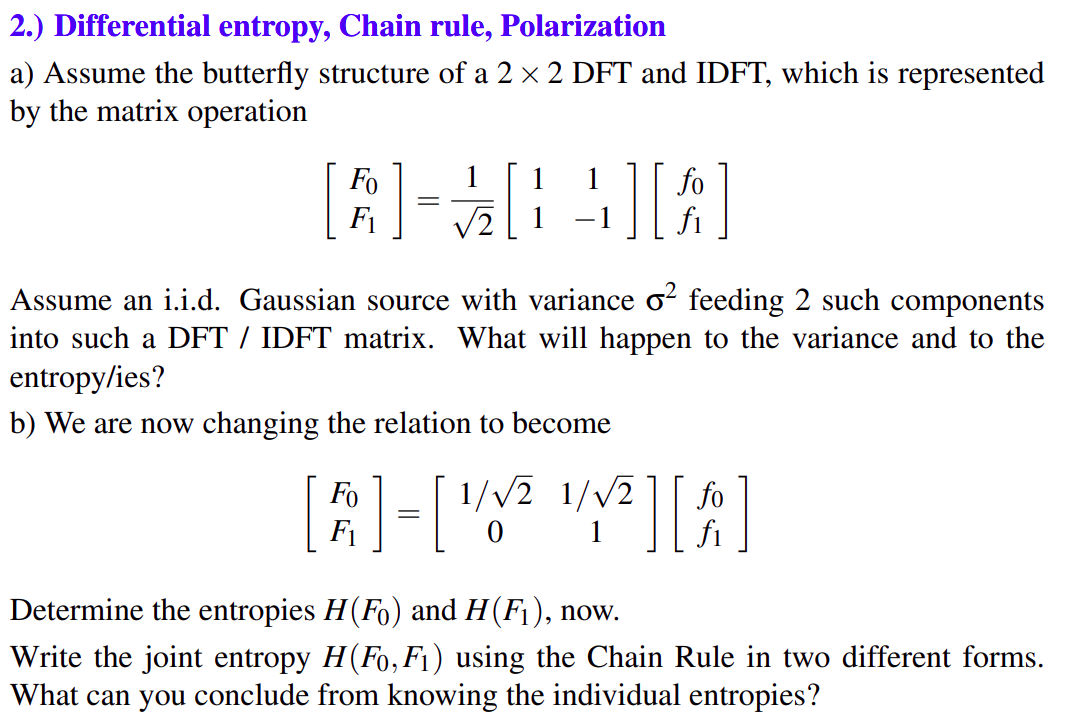
2.) Differential entropy, Chain rule, Polarization a) Assume the butterfly structure of a $2\times 2$ DFT and IDFT, which is represented by the matrix operation $\left[\begin{array}{l}{F}_0\\ F_1\end{array}\right]=\frac{1}{\sqrt{2}}\left[\begin{array}{cc}1& 1\\ 1& ?1\end{array}\right]\left[\begin{array}{l}{f}_0\\ f_1\end{array}\right]$ Assume an i.i.d. Gaussian source with variance ${?}^2$ feeding 2 such components into such a DFT / IDFT matrix. What will happen to the variance and to the entropy/ies? b) We are now changing the relation to become $\left[\begin{array}{l}{F}_0\\ F_1\end{array}\right]=\left[\begin{array}{cc}1/\sqrt{2}& 1/\sqrt{2}\\ 0& 1\end{array}\right]\left[\begin{array}{l}{f}_0\\ f_1\end{array}\right]$ Determine the entropies $H\left(F_0\right)$ and $H\left(F_1\right)$, now. Write the joint entropy $H\left(F_0,F_1\right)$ using the Chain Rule in two different forms. What can you conclude from knowing the individual entropies?