(Solved): 2. Air at the initial temperature \( T_{1}=25^{\circ} \mathrm{C} \) and pressure \( P_{1}=100 \mat ...

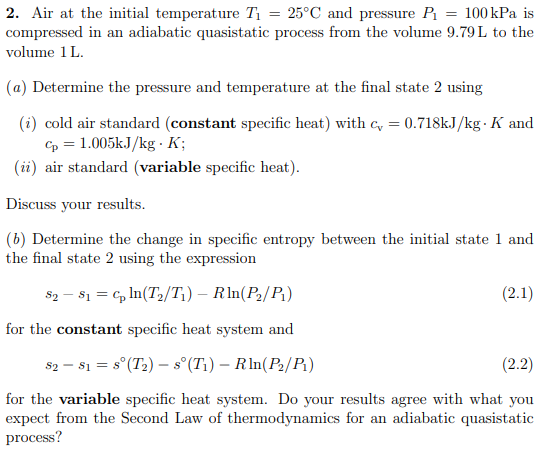
2. Air at the initial temperature \( T_{1}=25^{\circ} \mathrm{C} \) and pressure \( P_{1}=100 \mathrm{kPa} \) is compressed in an adiabatic quasistatic process from the volume \( 9.79 \mathrm{~L} \) to the volume \( 1 \mathrm{~L} \). (a) Determine the pressure and temperature at the final state 2 using (i) cold air standard (constant specific heat) with \( c_{\mathrm{v}}=0.718 \mathrm{~kJ} / \mathrm{kg} \cdot K \) and \( c_{\mathrm{p}}=1.005 \mathrm{~kJ} / \mathrm{kg} \cdot K \) (ii) air standard (variable specific heat). Discuss your results. (b) Determine the change in specific entropy between the initial state 1 and the final state 2 using the expression \[ s_{2}-s_{1}=c_{\mathrm{p}} \ln \left(T_{2} / T_{1}\right)-R \ln \left(P_{2} / P_{1}\right) \] for the constant specific heat system and \[ s_{2}-s_{1}=s^{\circ}\left(T_{2}\right)-s^{\circ}\left(T_{1}\right)-R \ln \left(P_{2} / P_{1}\right) \] for the variable specific heat system. Do your results agree with what you expect from the Second Law of thermodynamics for an adiabatic quasistatic process?