(Solved): 2-9 A flywheel of mass \( M \) is suspended in the horizontal plane by three wires of \( 1.829-\ma ...

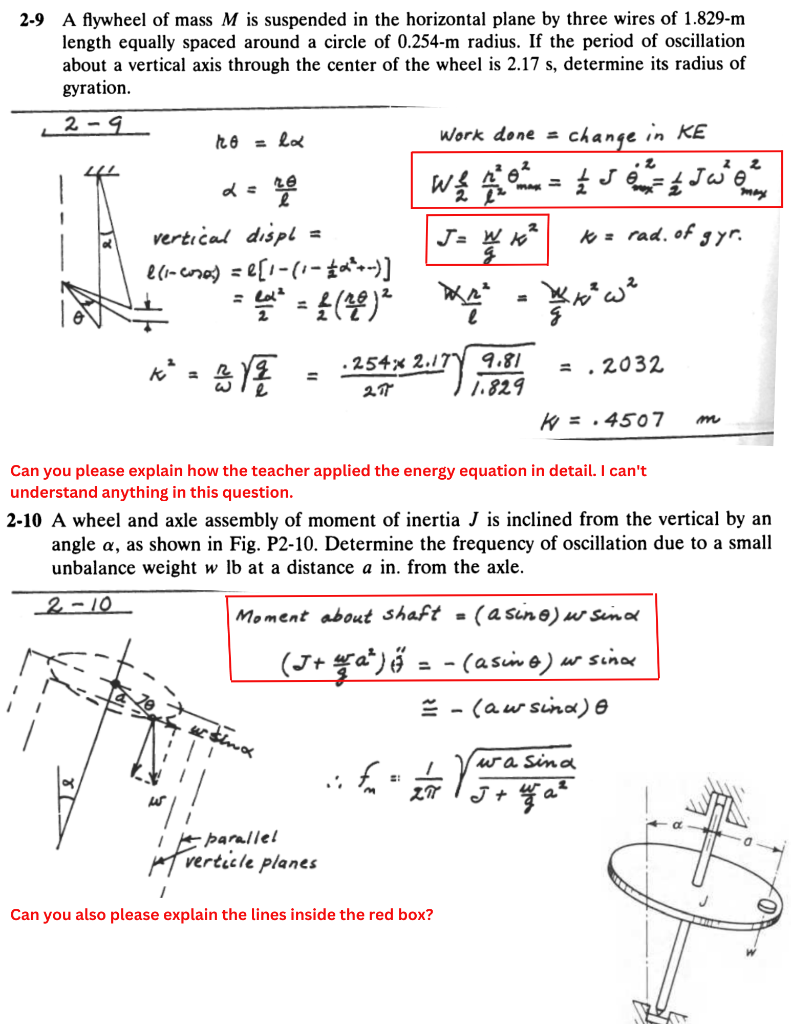
2-9 A flywheel of mass \( M \) is suspended in the horizontal plane by three wires of \( 1.829-\mathrm{m} \) length equally spaced around a circle of \( 0.254-\mathrm{m} \) radius. If the period of oscillation about a vertical axis through the center of the wheel is \( 2.17 \mathrm{~s} \), determine its radius of gyration. \[ \begin{array}{l} \frac{2-9}{k \theta}=l \alpha \quad \text { Work done }=\text { change in KE } \\ K^{2}=\frac{n}{\omega} \sqrt{\frac{g}{l}}=\frac{.254 \times 2.17}{2 \pi} \sqrt{\frac{9.81}{1.829}}=.2032 \\ F=.4507 \mathrm{~m} \\ \end{array} \] Can you please explain how the teacher applied the energy equation in detail. I can't understand anything in this question. 2-10 A wheel and axle assembly of moment of inertia \( J \) is inclined from the vertical by an angle \( \alpha \), as shown in Fig. P2-10. Determine the frequency of oscillation due to a small unbalance weight \( w \) lb at a distance \( a \) in. from the axle. \[ \begin{array}{l} t \text { about } \operatorname{shaft}=(a \sin \theta) \omega \sin \alpha \\ \left.T+\frac{w}{g} a^{2}\right) \theta^{\prime \prime}=-(a \sin \theta) w \sin \alpha \\ \cong-(a \omega \sin \alpha) \theta \\ \therefore f_{n}=\frac{1}{2 \pi} \sqrt{\frac{w a \sin \alpha}{\partial+\frac{w}{g} a^{2}}} \\ \text { es } \\ \end{array} \] Can you also please explain the lines inside the red box?