(Solved): 2) (40 pts.) For a one-dimensional harmonic oscillator with the potential \( V=\frac{1}{2} m \omeg ...

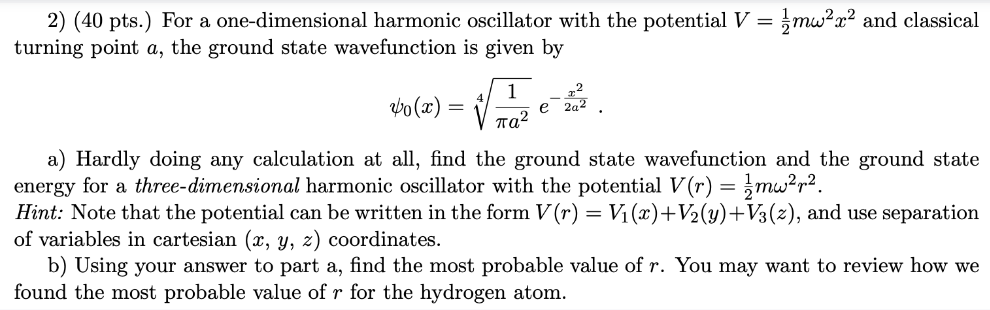
2) (40 pts.) For a one-dimensional harmonic oscillator with the potential \( V=\frac{1}{2} m \omega^{2} x^{2} \) and classical turning point \( a \), the ground state wavefunction is given by \[ \psi_{0}(x)=\sqrt[4]{\frac{1}{\pi a^{2}}} e^{-\frac{x^{2}}{2 a^{2}}} . \] a) Hardly doing any calculation at all, find the ground state wavefunction and the ground state energy for a three-dimensional harmonic oscillator with the potential \( V(r)=\frac{1}{2} m \omega^{2} r^{2} \). Hint: Note that the potential can be written in the form \( V(r)=V_{1}(x)+V_{2}(y)+V_{3}(z) \), and use separation of variables in cartesian \( (x, y, z) \) coordinates. b) Using your answer to part a, find the most probable value of \( r \). You may want to review how we found the most probable value of \( r \) for the hydrogen atom.