(Solved): 16/  A melting point test of \( n=10 \) samples of a binder used on manufacturing a rocket p ...

16/ 

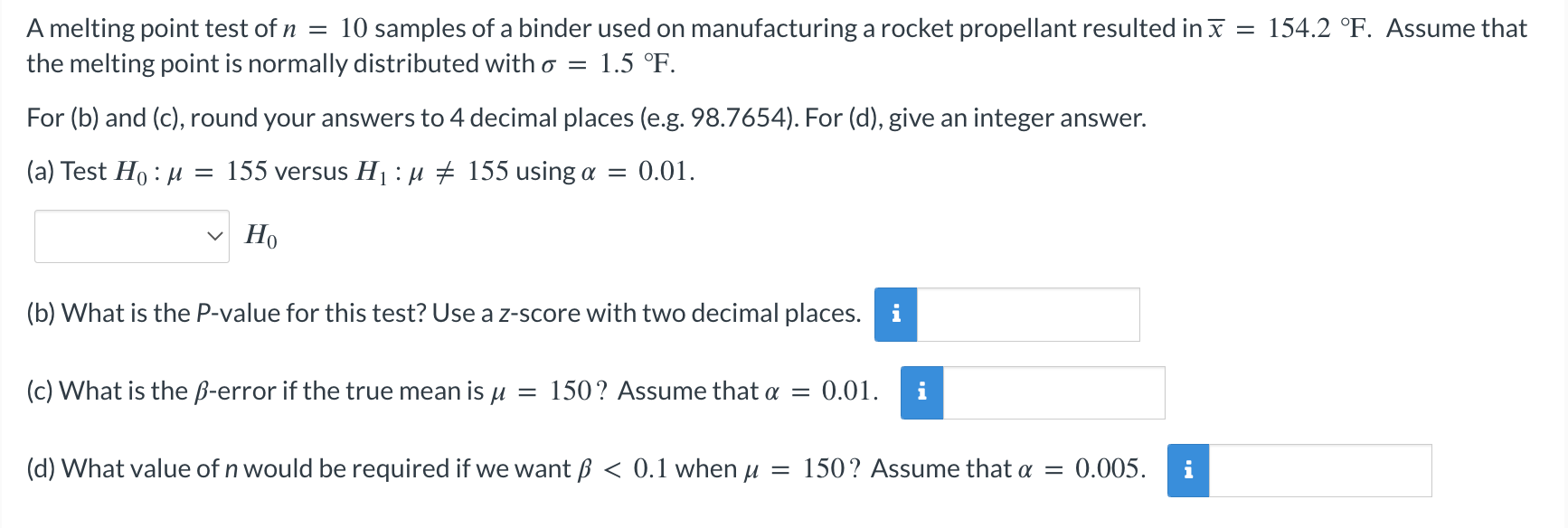
A melting point test of \( n=10 \) samples of a binder used on manufacturing a rocket propellant resulted in \( \bar{x}=154.2{ }^{\circ} \mathrm{F} \). Assume that the melting point is normally distributed with \( \sigma=1.5^{\circ} \mathrm{F} \). For (b) and (c), round your answers to 4 decimal places (e.g. 98.7654). For (d), give an integer answer. (a) Test \( H_{0}: \mu=155 \) versus \( H_{1}: \mu \neq 155 \) using \( \alpha=0.01 \). \[ \mathrm{H}_{0} \] (b) What is the \( P \)-value for this test? Use a z-score with two decimal places. (c) What is the \( \beta \)-error if the true mean is \( \mu=150 \) ? Assume that \( \alpha=0.01 \). (d) What value of \( n \) would be required if we want \( \beta<0.1 \) when \( \mu=150 \) ? Assume that \( \alpha=0.005 \).