(Solved): 152a. The zero-state scaled network shown in Figure \( 152 \mathrm{a} \) is driven by the current ...

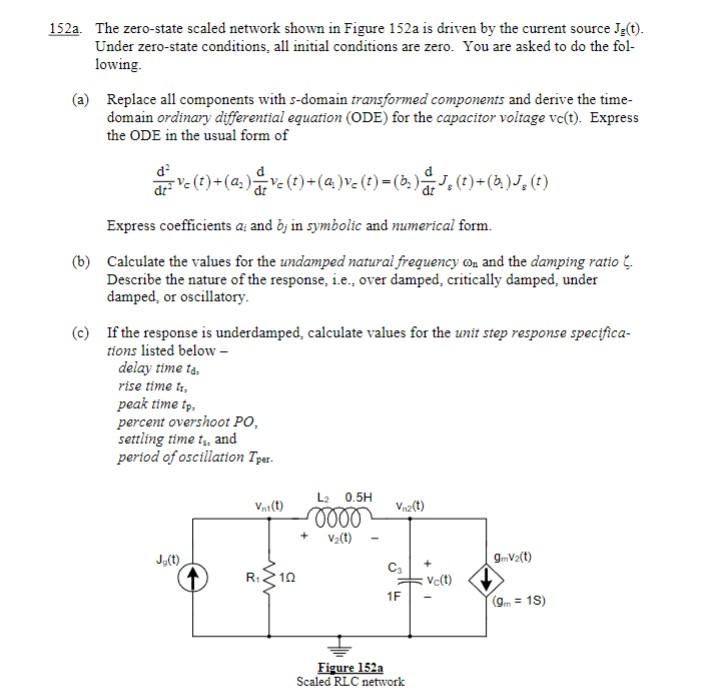
152a. The zero-state scaled network shown in Figure \( 152 \mathrm{a} \) is driven by the current source \( J_{\mathrm{g}}(\mathrm{t}) \). Under zero-state conditions, all initial conditions are zero. You are asked to do the following. (a) Replace all components with s-domain transformed components and derive the timedomain ordinary differential equation (ODE) for the capacitor voltage \( \mathrm{vc}(\mathrm{t}) \). Express the ODE in the usual form of \[ \frac{\mathrm{d}^{2}}{\mathrm{~d} t^{2}} v_{c}(t)+\left(a_{2}\right) \frac{\mathrm{d}}{\mathrm{d} t} v_{c}(t)+\left(a_{1}\right) v_{c}(t)=\left(b_{2}\right) \frac{\mathrm{d}}{\mathrm{d} t} J_{\varepsilon}(t)+\left(b_{1}\right) J_{s}(t) \] Express coefficients \( a_{i} \) and \( b_{j} \) in symbolic and numerical form. (b) Calculate the values for the undamped natural frequency \( \omega_{\mathrm{n}} \) and the damping ratio . Describe the nature of the response, i.e., over damped, critically damped, under damped, or oscillatory. (c) If the response is underdamped, calculate values for the unit step response specifications listed below - delay time \( t_{\mathrm{d}} \), rise time \( i_{\mathrm{r},} \) peak time \( t_{\mathrm{p}} \), percent overshoot \( P O \), settling time \( t_{\mathrm{l}} \) and period of oscillation \( T_{\text {per. }} \).