(Solved): 11. Chemotaxis of a cell in a microfluidics tube. Some cells have the ability to undergo chemotax ...

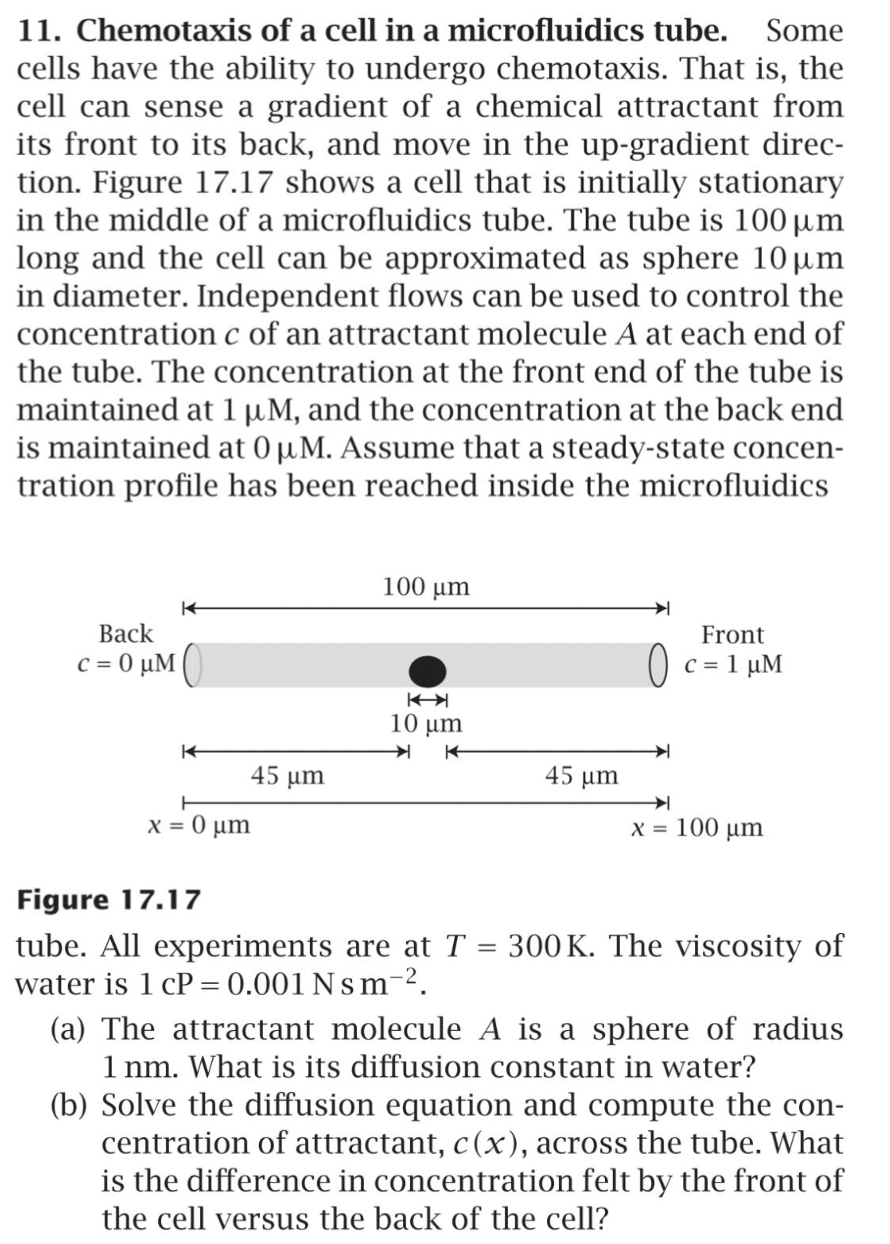
11. Chemotaxis of a cell in a microfluidics tube. Some cells have the ability to undergo chemotaxis. That is, the cell can sense a gradient of a chemical attractant from its front to its back, and move in the up-gradient direction. Figure 17.17 shows a cell that is initially stationary in the middle of a microfluidics tube. The tube is $100?\mathrm{m}$ long and the cell can be approximated as sphere $10?\mathrm{m}$ in diameter. Independent flows can be used to control the concentration $c$ of an attractant molecule $A$ at each end of the tube. The concentration at the front end of the tube is maintained at $1?\mathrm{M}$, and the concentration at the back end is maintained at $0?\mathrm{M}$. Assume that a steady-state concentration profile has been reached inside the microfluidics Figure 17.17 tube. All experiments are at $T=300\mathrm{K}$. The viscosity of water is $1\mathrm{cP}=0.001\mathrm{N}\mathrm{s}{\mathrm{m}}^{?2}$. (a) The attractant molecule $A$ is a sphere of radius $1\mathrm{nm}$. What is its diffusion constant in water? (b) Solve the diffusion equation and compute the concentration of attractant, $c(x)$, across the tube. What is the difference in concentration felt by the front of the cell versus the back of the cell? (c) Upon binding to a receptor on the cell surface, attractant $A$ triggers phosphorylation of another protein, $B$, at the front of the cell. Assume that $B$ can freely diffuse in the cytoplasm, and does so with a diffusion constant $D=10^{?10}{\mathrm{m}}^2{\mathrm{s}}^{?1}$. How long does it take for the phosphorylated protein $B$ to diffuse to the back end of the cell? (d) Now, instead assume that $B$ is a transmembrane protein, and therefore can only diffuse in the membrane (the protein is limited to the surface of the cell). It does so with a diffusion constant $D=10^{?11}{\mathrm{m}}^2{\mathrm{s}}^{?1}$. How long does it take now for $B$ to diffuse to the back end of the cell? Hint: Think about distances on a globe, rather than a straight-line distance.