Home /
Expert Answers /
Calculus /
10-points-use-the-error-formulas-to-find-bounds-for-the-error-when-int-0-1-x-1-dx-is-approxi-pa318
(Solved): (10 points) Use the error formulas to find bounds for the error when \int_0 (1)/(x+1)dx is approxi ...
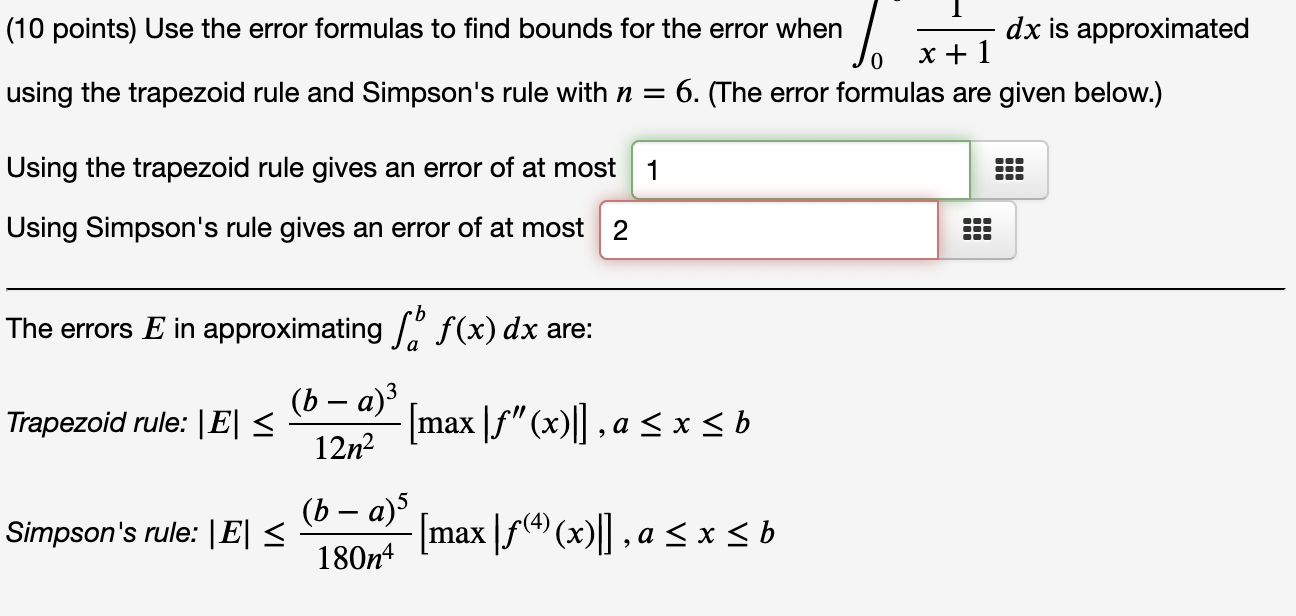
(10 points) Use the error formulas to find bounds for the error when
\int_0 (1)/(x+1)dxis approximated using the trapezoid rule and Simpson's rule with
n=6. (The error formulas are given below.) Using the trapezoid rule gives an error of at most Using Simpson's rule gives an error of at most The errors
Ein approximating
\int_a^b f(x)dxare: Trapezoid rule:
|E|<=((b-a)^(3))/(12n^(2))[max|f^('')(x)|],a<=x<=bSimpson's rule:
|E|<=((b-a)^(5))/(180n^(4))[max|f^((4))(x)|],a<=x<=b