(Solved): 1. Use the convolution integral to compute the convolution of \( y(t)=x(t) * h(t) \) for each pair ...

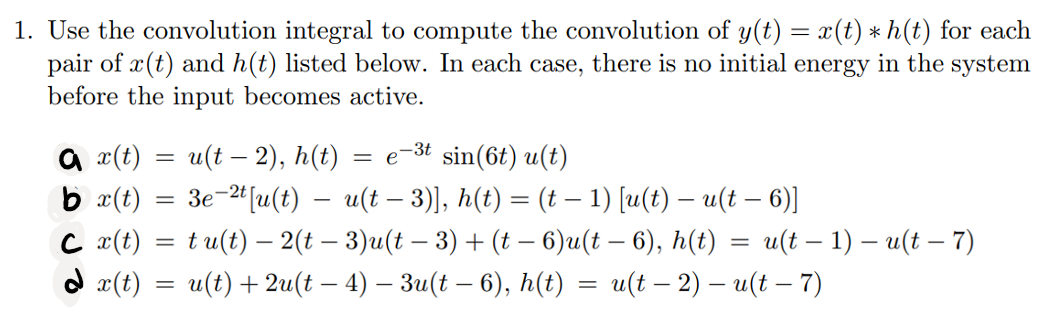
1. Use the convolution integral to compute the convolution of \( y(t)=x(t) * h(t) \) for each pair of \( x(t) \) and \( h(t) \) listed below. In each case, there is no initial energy in the system before the input becomes active. a \( x(t)=u(t-2), h(t)=e^{-3 t} \sin (6 t) u(t) \) b \( x(t)=3 e^{-2 t}[u(t)-u(t-3)], h(t)=(t-1)[u(t)-u(t-6)] \) C \( x(t)=t u(t)-2(t-3) u(t-3)+(t-6) u(t-6), h(t)=u(t-1)-u(t-7) \) d \( x(t)=u(t)+2 u(t-4)-3 u(t-6), h(t)=u(t-2)-u(t-7) \)