(Solved): (1 point) The figure shows level curves of a function \( f(x, y) \). (a) Draw gradient vectors at ...

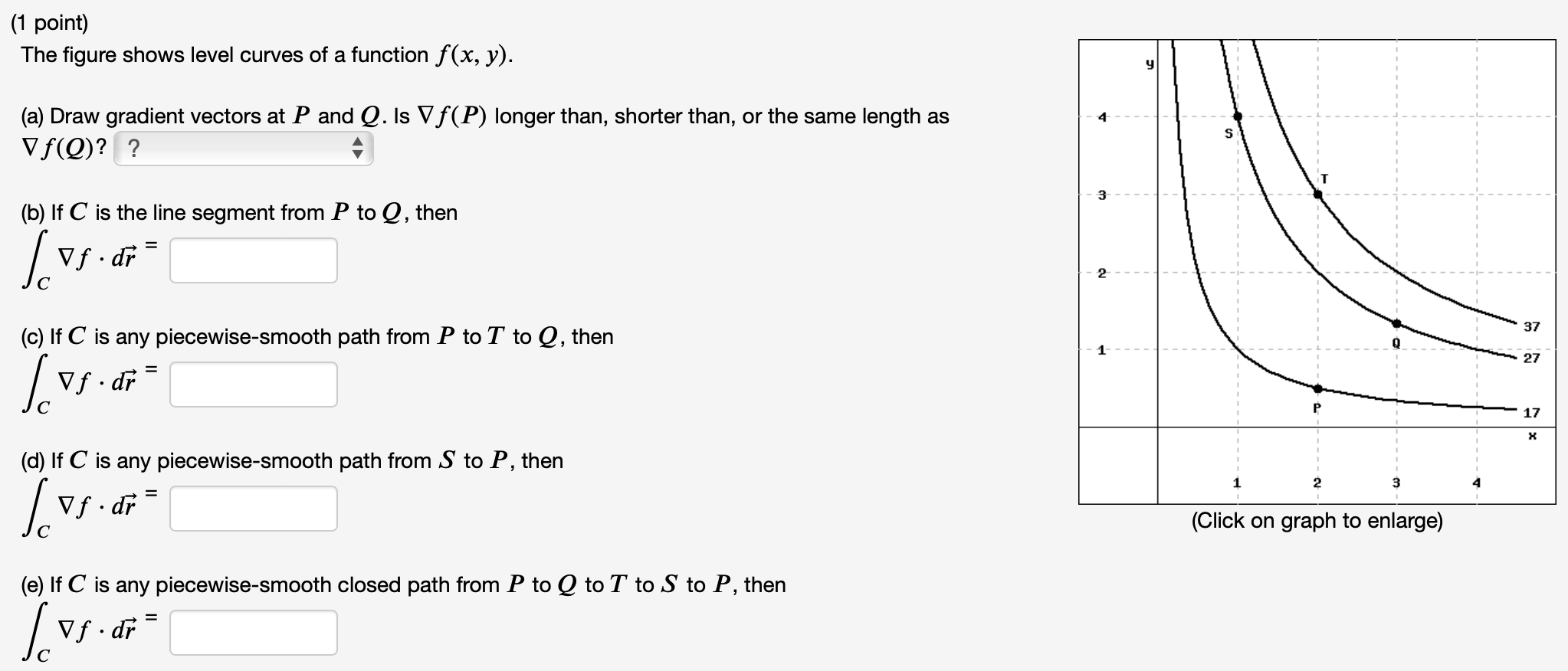
(1 point) The figure shows level curves of a function \( f(x, y) \). (a) Draw gradient vectors at \( P \) and \( Q \). Is \( \nabla f(P) \) longer than, shorter than, or the same length as \( \nabla f(Q) ? \) (b) If \( C \) is the line segment from \( P \) to \( Q \), then \[ \int_{C} \nabla f \cdot d \vec{r}= \] (c) If \( C \) is any piecewise-smooth path from \( P \) to \( T \) to \( Q \), then \[ \int_{C} \nabla f \cdot d \vec{r}= \] (d) If \( C \) is any piecewise-smooth path from \( S \) to \( P \), then \[ \int_{C} \nabla f \cdot d \vec{r}= \] (e) If \( C \) is any piecewise-smooth closed path from \( P \) to \( Q \) to \( T \) to \( S \) to \( P \), then \[ \int_{C} \nabla f \cdot d \vec{r}= \]