(Solved): 1. In this problem we introduce the concept of the determinant det(A) of a square matrix A. First, ...

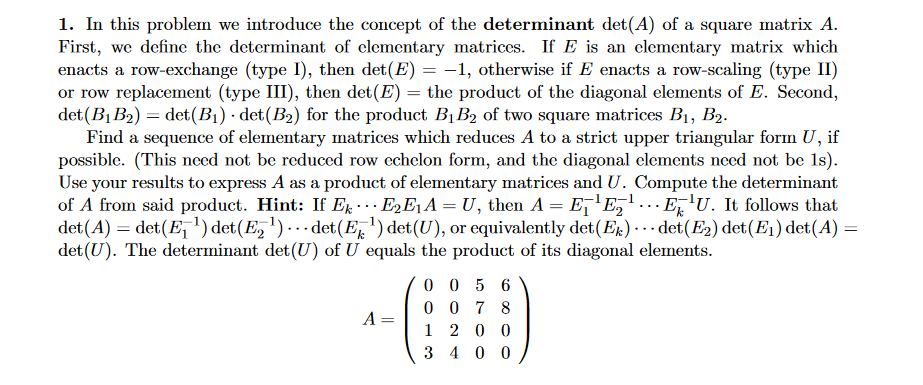
1. In this problem we introduce the concept of the determinant det(A) of a square matrix A. First, we define the determinant of elementary matrices. If E is an elementary matrix which enacts a row-exchange (type I), then det(E)=?1, otherwise if E enacts a row-scaling (type II) or row replacement (type III), then det(E)= the product of the diagonal elements of E. Second, det(B1?B2?)=det(B1?)?det(B2?) for the product B1?B2? of two square matrices B1?,B2?. Find a sequence of elementary matrices which reduces A to a strict upper triangular form U, if possible. (This need not be reduced row echelon form, and the diagonal elements need not be 1s). Use your results to express A as a product of elementary matrices and U. Compute the determinant of A from said product. Hint: If Ek??E2?E1?A=U, then A=E1?1?E2?1??Ek?1?U. It follows that det(A)=det(E1?1?)det(E2?1?)?det(Ek?1?)det(U), or equivalently det(Ek?)?det(E2?)det(E1?)det(A)= det(U). The determinant det(U) of U equals the product of its diagonal elements. A=???0013?0024?5700?6800????