(Solved): 1. Find a differential 0-form whose derivative d is the differential 1-form d = (5 + 8x)/ (1 ...

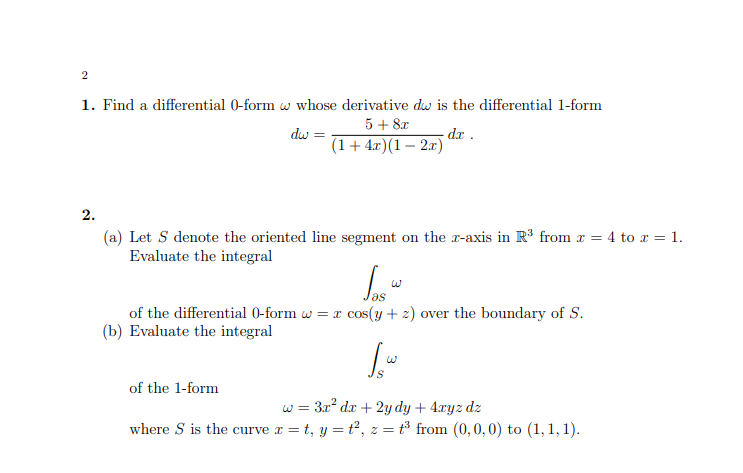
1. Find a differential 0-form ? whose derivative d? is the differential 1-form d? = (5 + 8x)/ (1 + 4x)(1 ? 2x) dx .

1. Find a differential 0 -form \( \omega \) whose derivative \( d \omega \) is the differential 1-form \[ d \omega=\frac{5+8 x}{(1+4 x)(1-2 x)} d x . \] 2. (a) Let \( S \) denote the oriented line segment on the \( x \)-axis in \( \mathbb{R}^{3} \) from \( x=4 \) to \( x=1 \). Evaluate the integral \[ \int_{\partial S} \omega \] of the differential 0 -form \( \omega=x \cos (y+z) \) over the boundary of \( S \). (b) Evaluate the integral \[ \int_{S} \omega \] of the 1-form \[ \omega=3 x^{2} d x+2 y d y+4 x y z d z \] where \( S \) is the curve \( x=t, y=t^{2}, z=t^{3} \) from \( (0,0,0) \) to \( (1,1,1) \).