(Solved): 1. Determine the Nyquist rate of the following signals: (a) x1(t)=2sinc10(420t) (b) x2(t)= ...

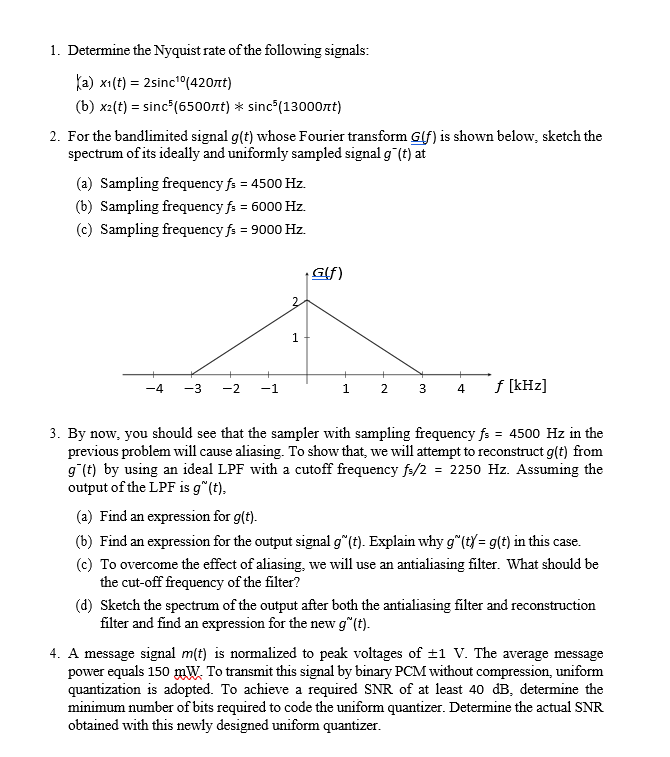
1. Determine the Nyquist rate of the following signals: (a) $$x_1(t)=2{\mathrm{sinc}}^{10}(420?t)$$ (b) $$x_2(t)={\mathrm{sinc}}^5(6500?t)?{\mathrm{sinc}}^5(13000?t)$$ 2. For the bandlimited signal $$g(t)$$ whose Fourier transform $$\underline{G}(f)$$ is shown below, sketch the spectrum of its ideally and uniformly sampled signal $$g^{?}(t)$$ at (a) Sampling frequency $$f_5=4500\mathrm{Hz}$$. (b) Sampling frequency $$f_s=6000\mathrm{Hz}$$. (c) Sampling frequency $$f_5=9000\mathrm{Hz}$$. 3. By now, you should see that the sampler with sampling frequency $$f_{\mathrm{s}}=4500\mathrm{Hz}$$ in the previous problem will cause aliasing. To show that, we will attempt to reconstruct $$g(t)$$ from $$g^{?}(t)$$ by using an ideal LPF with a cutoff frequency $$f_{\mathrm{s}}/2=2250\mathrm{Hz}$$. Assuming the output of the LPF is $$g^{?}(t)$$, (a) Find an expression for $$g(t)$$. (b) Find an expression for the output signal $$g^{?}(t)$$. Explain why $$g^N(t)=g(t)$$ in this case. (c) To overcome the effect of aliasing, we will use an antialiasing filter. What should be the cut-off frequency of the filter? (d) Sketch the spectrum of the output after both the antialiasing filter and reconstruction filter and find an expression for the new $$g^N(t)$$. 4. A message signal $$m(t)$$ is normalized to peak voltages of $$\pm 1$$ V. The average message power equals $$150\mathrm{mW}$$. To transmit this signal by binary PCM without compression, uniform quantization is adopted. To achieve a required SNR of at least $$40\mathrm{dB}$$, determine the minimum number of bits required to code the uniform quantizer. Determine the actual SNR obtained with this newly designed uniform quantizer.