(Solved): (1) Consider the vector forces in Figure 3. Please draw forces \( \vec{W}, \vec{X}, \vec{Y} \), an ...

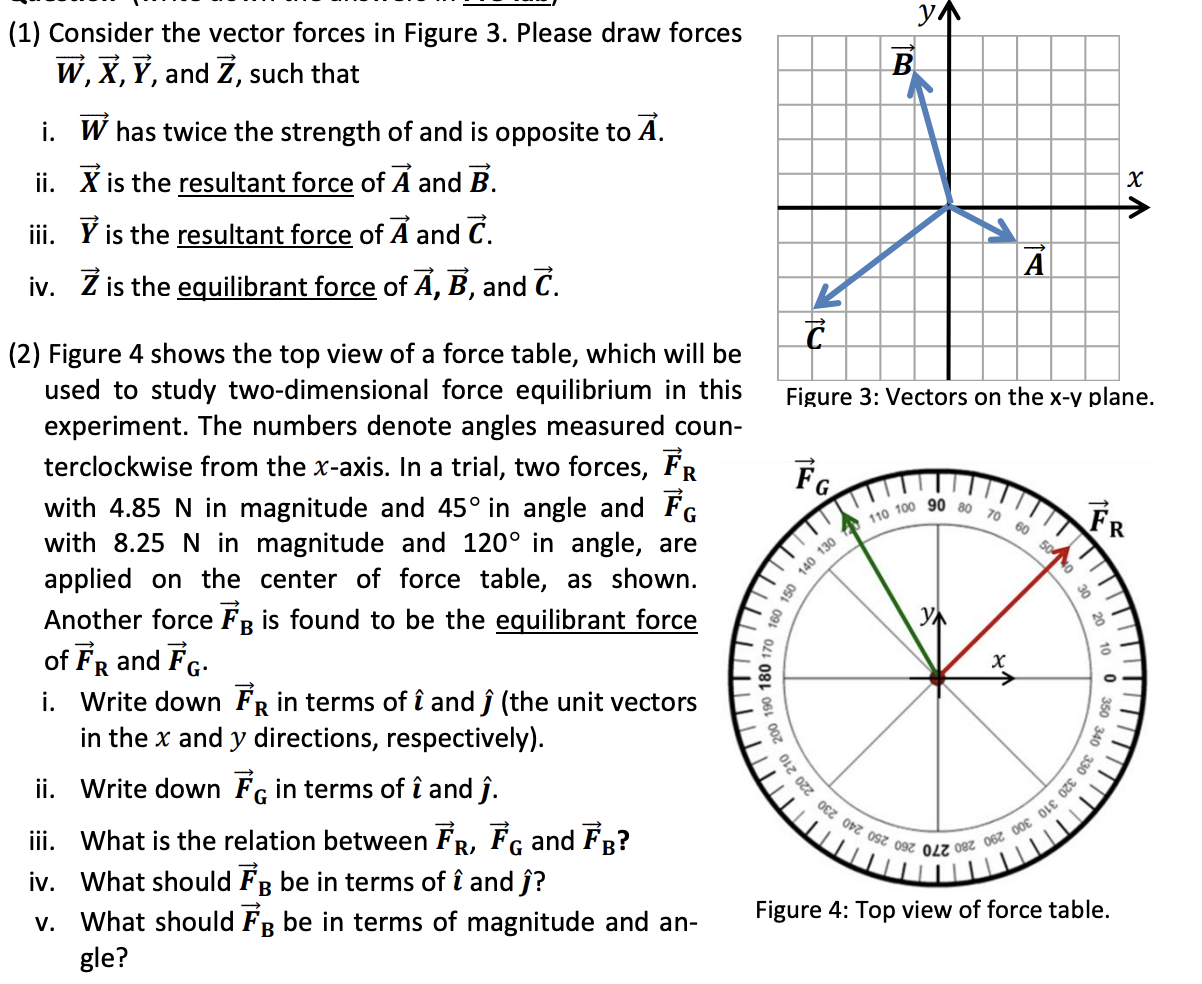
(1) Consider the vector forces in Figure 3. Please draw forces \( \vec{W}, \vec{X}, \vec{Y} \), and \( \vec{Z} \), such that i. \( \vec{W} \) has twice the strength of and is opposite to \( \vec{A} \). ii. \( \overrightarrow{\boldsymbol{X}} \) is the resultant force of \( \overrightarrow{\boldsymbol{A}} \) and \( \overrightarrow{\boldsymbol{B}} \). iii. \( \overrightarrow{\boldsymbol{Y}} \) is the resultant force of \( \vec{A} \) and \( \vec{C} \). iv. \( \overrightarrow{\boldsymbol{Z}} \) is the equilibrant force of \( \vec{A}, \vec{B} \), and \( \vec{C} \). (2) Figure 4 shows the top view of a force table, which will be used to study two-dimensional force equilibrium in this experiment. The numbers denote angles measured counterclockwise from the \( x \)-axis. In a trial, two forces, \( \overrightarrow{\boldsymbol{F}}_{\mathbf{R}} \) with \( 4.85 \mathrm{~N} \) in magnitude and \( 45^{\circ} \) in angle and \( \overrightarrow{\boldsymbol{F}}_{\mathbf{G}} \) with \( 8.25 \mathrm{~N} \) in magnitude and \( 120^{\circ} \) in angle, are applied on the center of force table, as shown. Another force \( \overrightarrow{\boldsymbol{F}}_{\mathbf{B}} \) is found to be the equilibrant force of \( \vec{F}_{\mathrm{R}} \) and \( \overrightarrow{\boldsymbol{F}}_{\mathrm{G}} \). i. Write down \( \overrightarrow{\boldsymbol{F}}_{\mathbf{R}} \) in terms of \( \hat{\boldsymbol{\imath}} \) and \( \hat{\boldsymbol{\jmath}} \) (the unit vectors in the \( x \) and \( y \) directions, respectively). ii. Write down \( \overrightarrow{\boldsymbol{F}}_{\mathrm{G}} \) in terms of \( \hat{\boldsymbol{\imath}} \) and \( \hat{\boldsymbol{\jmath}} \). iii. What is the relation between \( \vec{F}_{\mathrm{R}}, \overrightarrow{\boldsymbol{F}}_{\mathrm{G}} \) and \( \overrightarrow{\boldsymbol{F}}_{\mathrm{B}} \) ? iv. What should \( \overrightarrow{\boldsymbol{F}}_{\mathbf{B}} \) be in terms of \( \hat{\boldsymbol{\imath}} \) and \( \hat{\boldsymbol{\jmath}} \) ? v. What should \( \overrightarrow{\boldsymbol{F}}_{\mathbf{B}} \) be in terms of magnitude and an- Figure 4: Top view of force table. gle?