(Solved): 1. Consider a composite slabs consisting of two layers of different thicknesses and thermal con ...

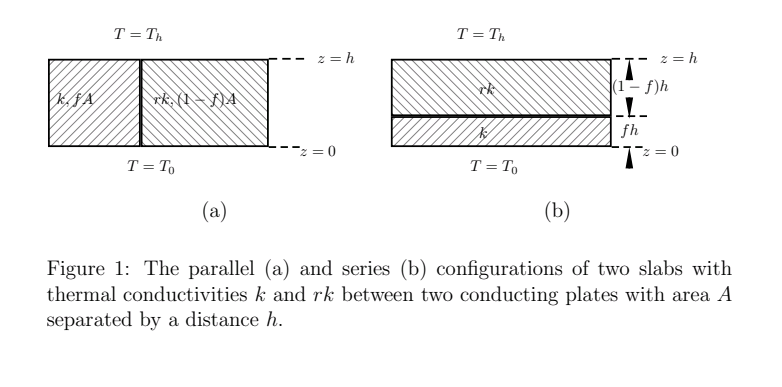
1. Consider a composite slabs consisting of two layers of different thicknesses and thermal conductivities shown in figure 4.3 (b). The thermal contact between the two layers is not perfect, and the temperatures in the two layers at the interface are different. The 'contact resistance' is defined as ${\mathcal{R}}_c=?\left(A?T/Q_z\right)$, where $?T$ is the difference in the temperatures of the two layers at the interface, $A$ is the cross-section area and $Q_z$ is the heat transfer rate. The thermal conductivities and thicknesses of the two slabs are $k_1=0.6\mathrm{W}/\mathrm{m}/{}^{?}\mathrm{C},k_2=2\mathrm{W}/\mathrm{m}/{}^{?}\mathrm{C},h_1=1\mathrm{cm}$, $h_2=1.5\mathrm{cm}$. When the temperature difference $T_h?T_0=80^{?}\mathrm{C}$, the flux is $q_z=3.2\times 10^3\mathrm{W}/{\mathrm{m}}^2$. What is the contact resistance? 2. The gap of thickness $h$ between two conducting plates of area $A$ and temperatures $T_0$ and $T_h$ can be filled by slabs of two materials, of thermal conductivities $k$ and $rk$, in two possible configurations shown in figure 1. Here, $r$ is the ratio of the conductivities of the two materials. In the parallel configuration, shown in 1 (a), the thickness of both slabs is $h$, and the cross sectional areas of the two slabs are $Af$ and $A(1?f)$ respectively, where $f<1$. In the series configuration, shown in 1 (b), the slabs have equal area, and thickness $fh$ and $(1?f)h$ respectively. Which configuration has a higher heat transfer rate? Hint: Write down the expression for the difference in the heat transfer rates in the two configurations, and examine whether this is positive or negative. (a) (b) Figure 1: The parallel (a) and series (b) configurations of two slabs with thermal conductivities $k$ and $rk$ between two conducting plates with area $A$ separated by a distance $h$.