(Solved): 1) As shown in Fig. 2, an incompressible and viscous fluid of density \( \rho \) and viscosity \( ...

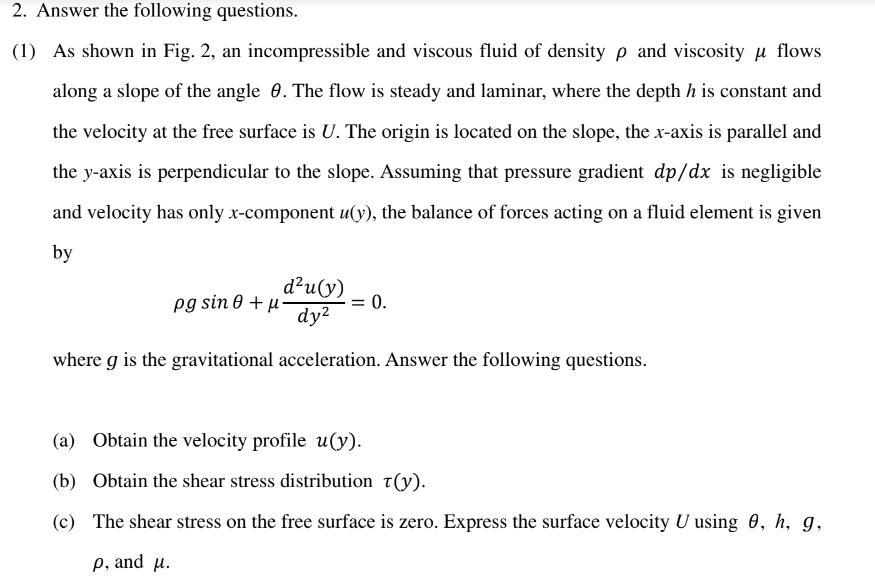
1) As shown in Fig. 2, an incompressible and viscous fluid of density \( \rho \) and viscosity \( \mu \) flows along a slope of the angle \( \theta \). The flow is steady and laminar, where the depth \( h \) is constant and the velocity at the free surface is \( U \). The origin is located on the slope, the \( x \)-axis is parallel and the \( y \)-axis is perpendicular to the slope. Assuming that pressure gradient \( d p / d x \) is negligible and velocity has only \( x \)-component \( u(y) \), the balance of forces acting on a fluid element is given by \[ \rho g \sin \theta+\mu \frac{d^{2} u(y)}{d y^{2}}=0 . \] where \( g \) is the gravitational acceleration. Answer the following questions. (a) Obtain the velocity profile \( u(y) \). (b) Obtain the shear stress distribution \( \tau(y) \). (c) The shear stress on the free surface is zero. Express the surface velocity \( U \) using \( \theta, h, g \), \( \rho \), and \( \mu \). (d) In the case that the volume flow rate per unit width \( Q \) is known, the fluid depth \( h \) is obtained by measuring the surface velocity \( U \). Express the fluid depth \( h \) by using \( Q \) and \( U \). Fig. 2 (2) Explain the nondimensional value which appears in a viscous term when the incompressible Navier-Stokes equations are nondimensionalized.