(Solved): 1 Area and Integrals Example 1. Use the geometry of the figures to find the areas of the shaded regi ...

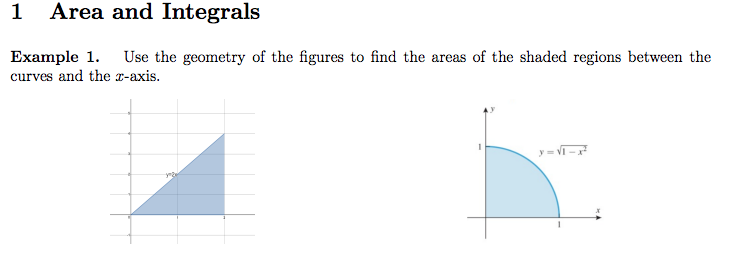
1 Area and Integrals Example 1. Use the geometry of the figures to find the areas of the shaded regions between the curves and the x-axis.

Example 2. Compute the net area between the function f(x) = 1 ? x 2 and the x-axis on the interval [0, 2].

is example 2 correct?

Example 1. Use the geometry of the figures to find the areas of the shaded regions between the curves and the \( x \)-axis. Example 2. Compute the net area between the function \( f(x)=1-x^{2} \) and the \( x \)-axis on the interval \( [0,2] \). \[ \begin{array}{l} \text { Net Area }=\int_{0}^{2}\left(1-x^{2}\right) d x=\left.\left[x-\frac{1}{3} x^{3}\right]\right|_{0} ^{2} \\ =\left[2-\frac{8}{3}\right]-[0-0] \\ =-\frac{2}{3} \\ \end{array} \]